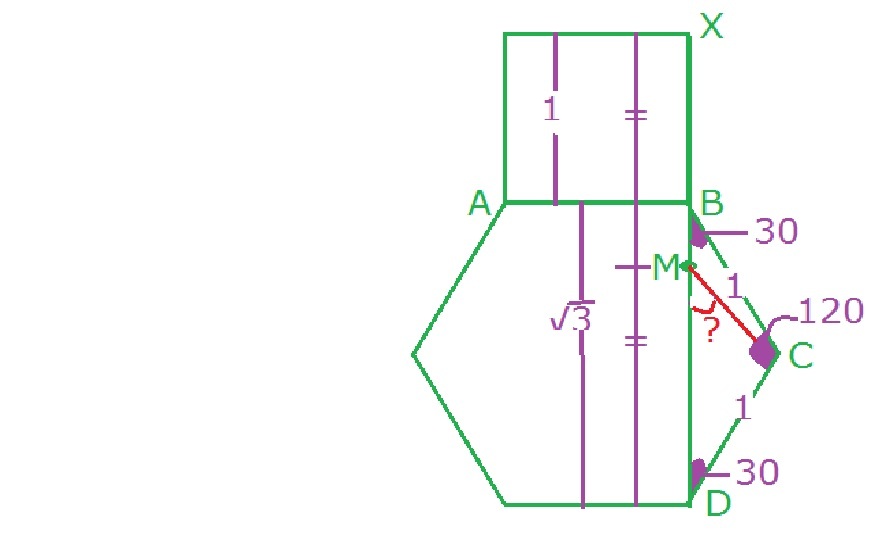
Square on a Hexagon

Since $ABCDEF$ is a regular hexagon, and $AXBY$ is a square, then $B$ lie on a line $DX$ . Suppose that $DC=a$ . Then $DB^2=DC^2+BC^2-2.DC.BC.\cos 120^{\circ}$ Means that $DB=a\sqrt{3}$ . So $DM=\dfrac{DB+BX}{2}=\dfrac{\sqrt{3}+1}{2}a$ Let $N$ be the midpoint of $DB$ . Then $DN=\dfrac{a\sqrt{3}}{2}$ . So $MN=DM-DN=\dfrac{a\sqrt{3}+a-a\sqrt{3}}{2}=\dfrac{a}{2}$ Since $CN=\dfrac{a}{2}$ , then $\angle CMD=\tan^{-1}\dfrac{CN}{MN}=\tan^{-1} 1=45^{\circ}$

Without loss of generality, let the side length of the hexagon be $2,$ and let the foot of the perpendicular from $C$ to $DX$ be $P.$ Then $\triangle BCP$ and $\triangle PCD$ are congruent $30-60-90$ right triangles, so $BP = PD = \sqrt{3}.$ We know that $BX = 2,$ so $DX = 2 + 2\sqrt{3}.$

Since $M$ is the midpoint of $\overline{DX},$ we have $DM = 1+\sqrt{3}.$ Since $PD = \sqrt{3},$ we have $PM = 1.$ Then $\triangle PMC$ is an isosceles right triangle. Therefore, $\text{m}\angle CMD = \text{m}\angle PMC = \boxed{45^{\circ}}.$

We let the side of the hexagon be $1$ . We drop the perpendicular from C to BD, and let the projection from C to BD be K. Since BC=CD, $\angle CBD=\angle CDB$ , so $\angle BCK=\angle DCK=\frac{120^\circ}{2}=60^\circ$ . Thus $BK=sin(60^\circ)BC=\frac{\sqrt3}{2}(1)=\frac{\sqrt3}{2}$ . Also, $CK=cos(60^\circ)BC=\frac{1}{2}(1)=\frac{1}{2}$ . Similarly $DK=\frac{\sqrt3}{2}$ , so $XD=1+2\frac{\sqrt3}{2}=1+\sqrt3$ . Hence $DM=\frac{1+\sqrt3}{2}$ , so $KM=\frac{\sqrt3+1}{2}-\frac{\sqrt3}{2}=\frac{1}{2}=CK$ . Thus $\angle CMK=\angle CMD=\angle MCK$ . Since $\angle CMK+\angle KCM=180^\circ-\angle CKM=180^\circ-90^\circ=90^\circ$ , $\angle CMK=\angle CMD=\frac{90^\circ}{2}=\boxed{45^\circ}$ .

Let the midpoint of $\overline{BD}$ be $N$ . Let $CN=x$ .

Since $ABCDEF$ is a hexagon, $\triangle DCN$ and $\triangle BCN$ are 30-60-90. Therefore, $BC=2x$ and $BN=ND=x\sqrt{3}$ . Since $ABCDEF$ and $ABXY$ have the same side length, $BX=2x$ . Therefore, $DX=2x\sqrt{3}+2x$ , and so $DM=x\sqrt{3}+x$ . We subtract length $DN=x\sqrt{3}$ to get $MN=x$ . Therefore, since $m\angle MNC=90^\circ$ and $MN=x=NC$ , the answer is $m\angle CMD=45^\circ$ .

Without loss of generality, we may conveniently assume length of each side as 2. Let O be the centre of the regular hexagon. AD passes through O, is of length 4 and by Pythagoras DE^2 = 16 - 4 = 12 . So DX = \sqrt{12}. + 2 = 2(\sqrt{3}. + 1). Let M be the midpoint of DX, So DM =\sqrt{3}. + 1.
Let N be the foot of the perpendicular from C upon DE. Clearly DN = NE = \sqrt{3}.
and CN = \sqrt{4 -3}\ = 1. Thus MN = DM - DN = 1 = CN . Hence CNM is a right angled isosceles triangle and angle CMN (or CMD) = 45 degrees.

$WLOG~let~AB=1.\\ ~~~~\\ We~know~\Delta~BCD~is~1\!-\!120^o\!-\!1.\\ ~~~~\\ \therefore~~BD=\sqrt3~and~\angle s~CBD=BDC=30.\\ ~~~~\\ \angle s~ABD=ABC-CBD=120-30=90.~\implies~XBD~is~a~st. line.\\ ~~~~\\ \therefore~XD=XB+BD=1+\sqrt3,~~\implies~MD=\frac 1 2 (1+\sqrt3).\\ ~~~~\\ In~~\Delta~MDC:-\\ ~~~~\\ Using~Cos~Rule~to~find~length ~of~MC.\\ ~~~~\\ MC^2=MD^2+DC^2 - 2*MD*DC*CosBDC\\ ~~~~\\ =(\frac 1 2( 1+\sqrt3))^2+1^2-2*\frac 1 2( 1+\sqrt3)*1*\dfrac{ \sqrt3} 2.\\ ~~~~\\ =\dfrac 1 2~~~\implies~MC=\sqrt{\frac 1 2}.\\ ~~~~\\ Using~Sin~Rule~to~find~\angle~CMD,\\ ~~~~\\ \dfrac{SinMDC}{MC} =\dfrac{SinCMD}1 \\ ~~~~\\ \implies ~SinCMD=\dfrac{\frac 1 2 }{\sqrt{\frac 1 2}} =\sqrt{\frac 1 2}. \\ ~~~~\\ \therefore~\angle~CMD=~~\color{#D61F06}{45^o}.$

use co-ordinate geometry. Take A as origin, AB as X axis. points X,B,D are co-linear. slope of MC =1 hence required angle=45 degree

Here are my steps:

1. Draw hexagon with square attached.
2. Extend the side XB of the square that intersects with two vertices of the hexagon. This line XD creates a triangle △BCD with two of the hexagon's sides. Let's assume the hexagon's side lengths are 1 unit.
3. Calculate the longer side of the triangle △BCD using trigonometry.
4. Add 1 unit to the calculated length because XB = BC = CD , XB = 1 unit.
5. Calculate midpoint M by dividing the line XD by 2. We now have a triangle △CMD .
6. We now know the length of line DM, the length of the line CD (1 unit), and the angle ∠CDM .
7. Use trigonometry to figure out the angle ∠CMD = 45˚.

First, note that $D$ , $B$ , and $X$ are colinear: since $ABCDEF$ is a regular hexagon, $DB$ is an altitude of the hexagon, and therefore is colinear with point $X$ since $\angle DBA + \angle ABX = \angle DBX$ , which is $90^{\circ} + 90^{\circ} = 180^{\circ}$ .

Without loss of generality, let $BC = 2$ . Now draw the center of the hexagon as point $O$ , draw equilateral triagles $BOC$ and $DOC$ , and let $BD$ intersect $OC$ at $K$ . We can see that $BD$ composes the altitudes of triangles $BOC$ and $DOC$ , and therefore, $BD = 2\sqrt{3}$ . Since $M$ is the midpoint of $DX$ , $DM$ has length $1 + \sqrt{3}$ . Also notice that $KM = DM - DK = (1 + \sqrt{3}) - (\sqrt{3}) = 1$ , so, since $CK = 1$ and $\angle CKM = 90^{\circ}$ , Triangle $CMK$ is a 45-45-90 degree triangle. So finally, since $\angle CMK = \angle CMD$ , $\angle CMD = \boxed{45^{\circ}}$ .