Squaring Pi

Geometry Level 1

Can the $\pi$ figure above, composed of 5 pieces of different colors, be rearranged into a perfect square?

The individual pieces can be rotated, reflected and translated.

Yes No

3 solutions

Andy Hayes
Apr 24, 2017

A possible solution is below:

how do you guys make these pictures?

Terry Yu - 4 years, 1 month ago

As far as I can determine, that is the unique solution (up to rotation / reflection).

Do you see another way?

Calvin Lin Staff - 4 years, 1 month ago

If you allow for flipping the pieces upside-down, then this results in distinct solutions.

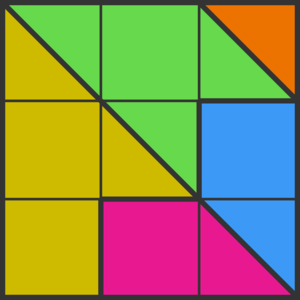Blue and Green pieces flipped upside-down

You can also switch the pink and blue pieces, since they are identical through rotation.

Andrew Hayes Staff - 4 years, 1 month ago

Yes, I too would like to know how these images are made.

Jesse Nieminen - 4 years, 1 month ago

@Jesse Nieminen – @Jesse Nieminen - The images are made on figma. Your welcome.

Terry Yu - 4 years, 1 month ago

@Terry Yu – Thank you!

Jesse Nieminen - 4 years, 1 month ago

That's interesting. here is a discussion that is sort of related.

Agnishom Chattopadhyay - 4 years, 1 month ago

Is in the question implicit that it has to be composed of 5 colors all the same? I don't think so, either way the answer is "yes".

Giovanna Andrade - 4 years, 1 month ago

McKenna Bassett
May 7, 2017

9 squares = 3 X 3

The black lines mean nothing other than the size of the objects I believe. I don't think this proves anything as the ability to put the colored pieces together is dependent on shape also.

Andrew Allen - 4 years, 1 month ago

If two collections of pieces have the same area, it doesn't mean that the other one can be constructed by rearranging the pieces of the first one. Hence, your solution is invalid.

Jesse Nieminen - 4 years, 1 month ago

It is a necessary condition of course, but it is not sufficient!

Andrea Virgillito - 4 years, 1 month ago

this doesn't prove it can be a square.....

Chimbuchi Kenneth Nwagu - 4 years, 1 month ago

What Andrew said.

Steve Powersuit - 4 years, 1 month ago

Eric Langley
May 13, 2017

You may have noticed that if you remove the very edges of the pi symbol, you have a perfect square left over (minus the two edges of course). Transfer those two edge squares to the empty space and you have your completed perfect square.

I'm not quite sure what you mean. You cannot choose how to cut the shape, but are restricted to the colored pieces as shown.

Calvin Lin Staff - 4 years ago

Squaring Pi

3 solutions

0 pending reports