Stop shouting! pt.2
a ! b ! = a ! + b ! + c ! , a + b + c = ?
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
S i n c e a a n d b a r e i n t e r c h a n g e a b l e , w e c a n s a f e l y a s s u m e a = b o r a > b . N o t e t h a t n ! > 0 , a n d s o m i n i m u m c ! = 1 . I f b = 1 , a ! ∗ b ! < a ! + b ! , ⟹ a = b > 1 I f a = b = 2 , a ! ∗ b ! = a ! + b ! + 0 , b u t c ! = 0 , ∴ a = b = 2 n o t a s o l u t i o n . 1 2 = 3 ! ∗ 2 ! = 3 ! + 2 ! + 4 . B u t c ! = 4 . n o s o l u t i o n . N e x t 3 ! ∗ 3 ! = 3 6 = 3 ! + 3 ! + 2 4 s o c ! = 2 4 a n d c = 4 . S o l u t i o n ( a , b , c ) = ( 3 , 3 , 4 ) o n l y . a + b + c = 3 + 3 + 4 = 1 0 .
Let a , b , c ∈ N ∪ { 0 } such that
a ! + b ! + c ! = a ! b ! . ( ∗ )
Notice that ( ∗ ) can be rewritten as c ! + 1 = ( a ! − 1 ) ( b ! − 1 ) . ( ∗ ∗ )
For a ≤ 1 or b ≤ 1 , ( ∗ ) becomes 1 + c ! = 0 , which is impossible for c ! > 0 .
WLOG, let b ≥ a ≥ 2 .
Case 1 : c ≤ b
Dividing ( ∗ ) by a ! and c ! yields
1 + a ! b ! + a ! c ! = b ! and 1 + c ! b ! + c ! a ! = c ! a ! b !
respectively. Both a ! b ! and c ! b ! are integers since b ≥ a and b ≥ c .
Therefore, the former and latter equalities imply c ≥ a and a ≥ c respectively, i.e. c = a .
Putting a = c into ( ∗ ∗ ) yields
c ! + 1 = ( c ! − 1 ) ( b ! − 1 ) ⟺ ( c ! − 1 ) + 2 = ( c ! − 1 ) ( b ! − 1 ) ⟺ 2 = ( c ! − 1 ) ( b ! − 2 )
So b ! − 2 is either 2 or 1 , i.e. either b ! = 4 or b ! = 3 , but such b cannot be found.
Case 2 : b < c
Dividing ( ∗ ) by a ! and b ! yields
1 + a ! b ! + a ! c ! = b ! and 1 + b ! a ! + b ! c ! = a !
respectively. Using the similar argument as Case 1 , we have a = b . Putting a = b into ( ∗ ) yields
c ! = b ! ( b ! − 2 ) ( ∗ ∗ ∗ )
Observe that C b c = b ! ( c − b ) ! c ! is an integer and now we have
C b c = b ! ( c − b ) ! c ! = ( c − b ) ! b ! − 2 .
Note that ( c − b ) ! b ! − 2 = C b c ≥ 1 . It follows that b ! ≥ ( c − b ) ! + 2 > ( c − b ) ! , and so b ≥ c − b .
Consequently, ( c − b ) ! b ! is an integer. By rewriting this result as
C b c = ( c − b ) ! b ! − 2 = ( c − b ) ! b ! − ( c − b ) ! 2 ,
we have ( c − b ) ! as a divisor of 2 . Since c > b , c − b is either 2 or 1 .
Sub-case 2 (a) :
For c = b + 1 , putting this result into ( ∗ ∗ ∗ ) yields b ! − b = 3 , for which b is a divisor of 3 . Hence b is either 1 or 3 , but only b = 3 satisfies this relation.
Sub-case 2 (b) :
For c = b + 2 , putting this result into ( ∗ ∗ ∗ ) yields b ! − b 2 − 3 b = 4 .
Clearly, b divides 4 and so b = 1 , 2 or 4 . However, none of them satisfy b ! − b 2 − 3 b = 4 .
In conclusion , a = b = 3 and c = 4 and so a + b + c = 1 0 .
That's funny. Only one word was underlined in this whole thing for being incorrect, and it was the word greek. Ha Ha!
for a=0:10
for b=0:10
for c=0:10
if (factorial(a)*factorial(b)==factorial(a)+factorial(b)+factorial(c))
a+b+c
end
end
end
end
Answer: 10
What if a , b , c > 1 0 ? Your solution will not found them.
Log in to reply
Yaa it is only to find the answer! I could not solve the problem.
Log in to reply
However good work. It's not forbidden to use PC ;)
Log in to reply
@Drop TheProblem – Yaa brute force. I would love to know the analytical way to solve.
Log in to reply
@Shib Shankar Sikder – I've given an 'analytical way to solve'.
If it is greater than 10, then he could re-write the program to account for that. Luckily, that was not a problem.
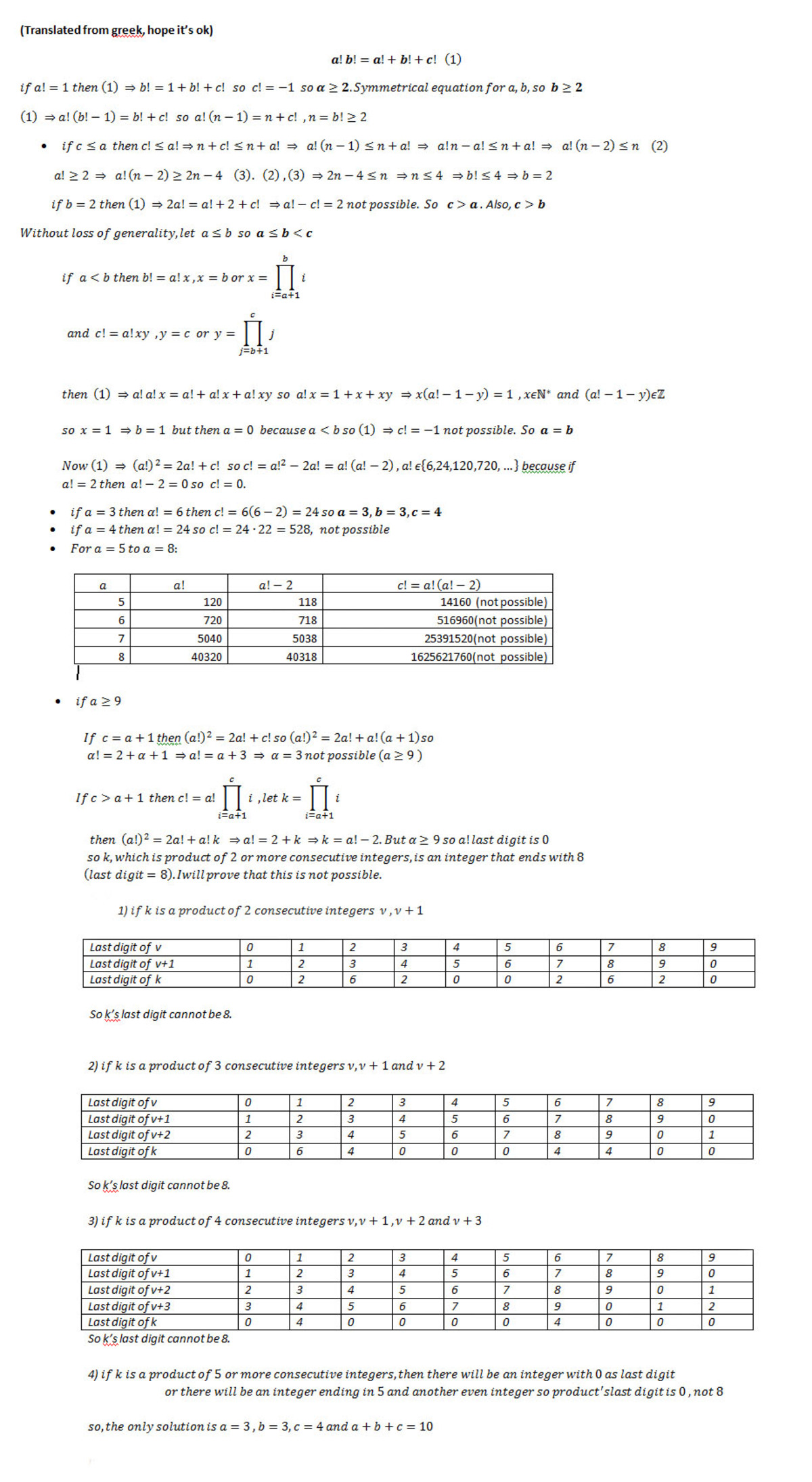
Wlog a ≥ b ≥ 2 (none of a , b can be 0 or 1 ). Then a = b + k with k ≥ 0
Then a ! = b ! a ! + 1 + b ! c ! implies c = b + m ≥ 2 with m ≥ 0 .
b ! = 1 + ( b + k ) ! b ! + ( b + k ) ! ( b + m ) ! . Two cases:
If k > 0 , then k > m ≥ 0 , so a > c ≥ b .
Then c ! a ! b ! = c ! a ! + c ! b ! + 1 implies b ≥ c , so b = c and a > b = c .
Then a ! ( b ! − 1 ) = 2 b ! , so a ! ∣ 2 b ! , so a ! ≤ 2 b ! ≤ ( b + 1 ) ! ⟹ a ≤ b + 1 and b + 1 ≥ a > b = c , so b + 1 = a .
( b + 1 ) ! b ! = ( b + 1 ) ! + 2 b ! ⟺ ( b + 1 ) ! = b + 3 , so b ∣ 3 ⟺ b ∈ { 1 , 3 } , none of which solve the last equation.
If k = 0 , then a = b . Then a ! 2 = 2 a ! + ( a + m ) ! ⟺ a ! = 2 + a ! ( a + m ) ! , so m ! ∣ a ! − 2 > 0 , since ( a a + m ) = a ! m ! ( a + m ) ! is a positive integer.
m ! ≤ a ! − 2 < a ! ⟹ m < a . But then m ! ∣ a ! , so m ! ∣ 2 ⟺ m ∈ { 0 , 1 , 2 } .
If m = 0 , then a = b = c ⟹ a ! 2 = 3 a ! ⟺ a ! = 3 , impossible.
If m = 2 , then a ! 2 = 2 a ! + ( a + 2 ) ( a + 1 ) a ! ⟺ a ! = a 2 + 3 a + 4 , so a ∣ 4 ⟺ a ∈ { 1 , 2 , 4 } . None of these solve the last equation.
If m = 1 , then a ! 2 = 2 a ! + ( a + 1 ) a ! ⟺ a ! = a + 3 , so a ∣ 3 ⟺ a ∈ { 1 , 3 } , which gives the only solution ( a , b , c ) = ( 3 , 3 , 4 ) .