Big Perfect Square
Find the smallest positive integer x such that 4 x + 4 5 5 5 + 4 6 6 6 is a positive integer.
The answer is 443.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
In fact:
4 4 4 3 + 4 5 5 5 + 4 6 6 6 = 2 4 4 3 1 + 4 1 1 2 + 4 2 2 3
1 + 4 1 1 2 + 4 2 2 3 = 1 + 2 2 2 4 + 4 2 2 3 = 1 + 2 × 2 2 2 3 + ( 2 2 ) 2 2 3 = 1 + 2 2 2 3
So, the expression equals 2 4 4 3 + 2 6 6 6 at x = 4 4 3 which means the answer x would satisfy x ≤ 4 4 3 .
Log in to reply
By the way, with wolframalpha, I found that:
4 6 6 6 + 4 5 5 5 − ( 2 6 6 6 + 2 4 4 3 − 2 2 1 9 + 2 − 4 ) ≈ − 5 . 7 9 5 6 × 1 0 − 6 9
New edit:
Also, by wolframalpha help, I found that 3 3 4 ≤ x ≤ 4 4 3
Log in to reply
How can you know it is 443 if you don't have calculator or wolframalpha?
Log in to reply
@Porames Vattanaprasan – I just stated x<=443, it could be lower but if you ask how 443 work, see above. I posted one solution.
Log in to reply
@Gian Sanjaya – Good luck my friend :)
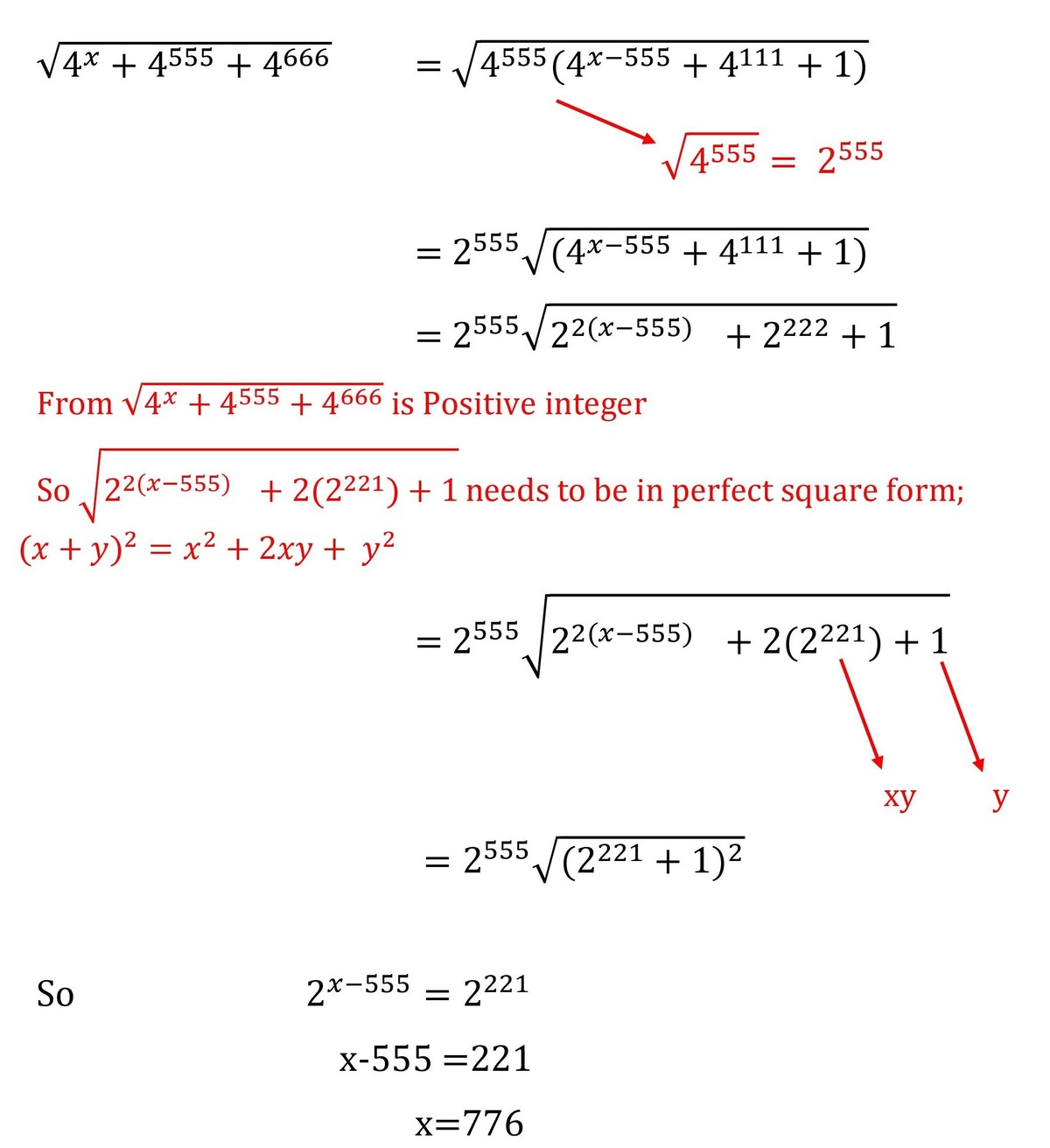

We want 4 x + 4 5 5 5 + 4 6 6 6 to take on a perfect square form, so we opt to rewrite it as 2 2 x + 2 ∗ 2 1 1 0 9 + 2 1 3 3 2 . If we try a factorization of ( 2 x + 2 6 6 6 ) ( 2 x + 2 6 6 6 ) , then x = 4 4 3 .