Tension in the string
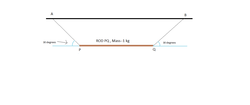 A uniform rod of mass
1
k
g
is hanging as shown.
A uniform rod of mass
1
k
g
is hanging as shown.
Find the tension in string A P (in newtons) immediately after string B Q is cut.
Assumption
∙ g = 9 . 8 m/s 2
The answer is 2.8.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
note- i neglected centripetal force because it is 0 immediately after cutting string because the rod begins with 0 angular velocity
Log in to reply
We have asked to find out just immediately after the string is cut. So at that instant, angular acceleration should be qero know..??... Can u plz explain??
Log in to reply
The angular acceleration is not zero immediately after the string is cut because there is a non zero torque about the centre ,, because the tension has instantaneously reduced to zero on one side when the string is cut but not on the other string,, In any any instant if net torque is not zero, the angular acceleration is non zero about the point too,
However,, you can surely say that the angular velocity was zero just after cutting because the system begins from rest , velocity is the quantity associated directly with the state of motion and not the angular acceleration. (as known from the first law of motion)
Log in to reply
@Mvs Saketh – Ohh yeah u r absolutely rite...i got confused about it... Anyways thanks for giving such an awesome explanation
Thank you for the solution. But I didn't get one part of it. Why the string being inextensible implies that the acceleration along it is zero. Will you please clarify it?
could you explain with a diagram how you got the equation of acceleration.
the net acceleration along the string is = v^2/r . but since just after the release the value of v = 0 , hence acceleration along the string is 0 . would have been a complete different situation if the problem had asked the tension after some time t or when the rod made an angle with the horizontal .
@Sumanth R Hegde y has he used +@l/2sin theta it should be - instead of plus right ?
Good question and an even better solution.Thanks for the solution...Coudn't solve this one :P.Messed up with the constraint part.Thanks for the solution again,now this mistake won't be repeated.
The constraint that I have imposed is only the acceleration of the point attached to the string along the string must be zero.Just after cutting let the tension be T .then resolving T into its components and taking the torque due to only the component perpendicular to the rod ( T / 2 ) ( L / 2 ) = M L 2 / 1 2 @ so @ = 3 T / M L .( @ is the angular acceleration of the body).now we observe that the acceleration of point considered is a ( y ) = ( T / 2 M + @ L / 2 − g ) in the upward direction and a ( x ) = ( T √ 3 / 2 M ) in horizontal.so using the constraint a ( x ) c o s 3 0 + a ( y ) s i n 3 0 = 0 .Now plugging the values of a ( x ) and a ( y ) we get T = 2 M g / 7
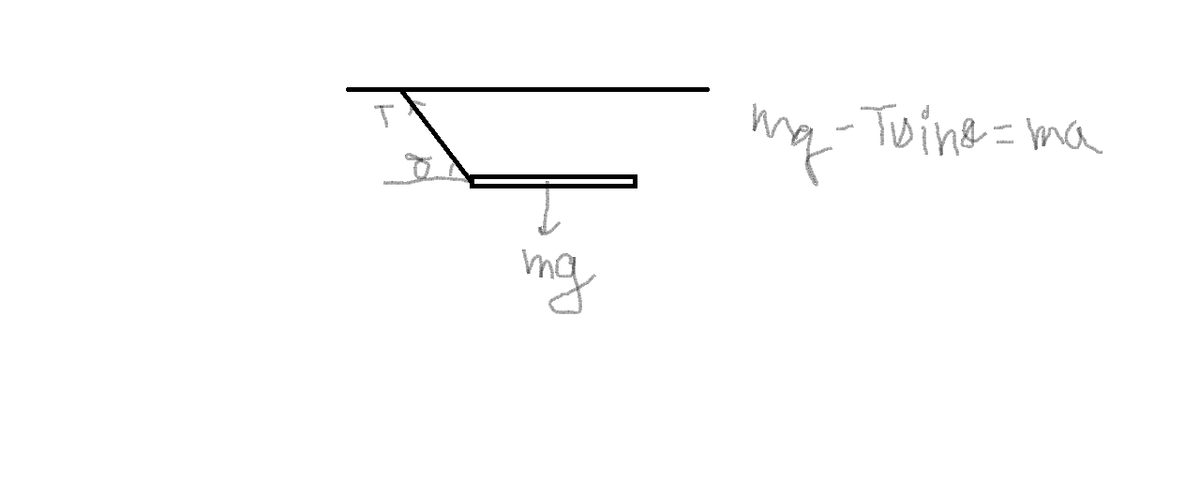 also write torques about c.o.m , you get the ans as 2.823 !
also write torques about c.o.m , you get the ans as 2.823 !
Consider the situation of the rod immediately after collision, make the following observations 1) centre of mass falls under gravity
2) Tension tends to oppose gravity and thus centre of mass does not free fall with acceleration 'g'
3) As the string is not normal to rod it has a non zero horizontal component which means centre of mass does not fall vertically downward
4) The string being inextensible (Constant length) means that as long as the string is not slack it will describe a circle about the pivot at the roof , thus the end of the rod just attached to the string describes an arc of the circle , thus moving PERPENDICULAR to the string.
5) And finally the torque about centre of mass is purely due to tension
Now lets state the above statements mathematically so that we can solve it
m g − T s i n ( θ ) = m a y − − − ( 1 ) T c o s ( θ ) = m a x − − − ( 2 ) a n d 2 l T s i n ( θ ) = I α − − − ( 3 ) N o w l e t s d e r i v e t h e c o n s t r a i n g e q u a t i o n A l l c o m p o n e n t s o n t h e r o d h a v e t h e f o l l o w i n g a c c e l e r a t i o n s
Now lets derive the constraing equation
any part of the rod experiences two accelerations,, one translation by virtue of com and one rotational by virtue of rotation about com
Thus the superposition of these two components gives the resultant acceleration at any point
For the end of rod just tied to string AP
the superposition of these accelerations give 0 net component along the string as the string is inextensible ,
the accelerations experienced by this part is
a x a l o n g h o r i z o n t a l a y a l o n g v e r t i c a l ( b y v i r t u e o f t r a n s l a t i o n o f c o m ) a n d α 2 l p e r p e n d i c u l a r t o r o d T h u s e q u a t i n g c o m p o n e n t s a l o n g s t r i n g t o 0 w e g e t a x c o s ( θ ) + α 2 l s i n ( θ ) − a y s i n ( θ ) = 0 − − − ( 4 )
Now solve these 4 equations to get
T = 3 ( s i n ( θ ) ) 2 + 1 m g s i n ( θ )
which on putting values you get the answer as 2.8