The Disc is retarded to 0 rad/s
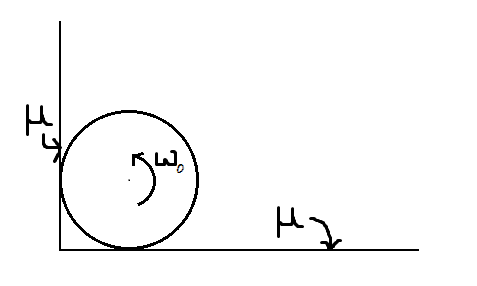 Consider a disc of mass
and radius
touching a rough wall of coefficient of friction
and a rough floor of coefficient of friction
as shown in figure. The ring has an initial angular velocity of
.
Consider a disc of mass
and radius
touching a rough wall of coefficient of friction
and a rough floor of coefficient of friction
as shown in figure. The ring has an initial angular velocity of
.
Find the time taken by the ring to come to a halt.
Details and Assumptions
-
The disc neither translates on the floor nor on the wall
-
-
-
-
-
Try my set
The answer is 3.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The first assumption tells us that the disc does not translate on either the wall or floor. We can hence consider translational equilibrium first.
∑ F x = ∑ F y = 0
Here I am denoting the frictional force and the normal contact force exerted by the wall as f 1 and N 1 respectively , frictional force and the normal contact force exerted by the floor as f 2 and N 2 respectively.
∑ F x = N 1 − f 2 = 0
∑ F y = f 1 + N 2 − m g = 0
f 1 = μ N 1 and f 2 = μ N 2
Analyzing the rotational motion ,
The frictional forces f 1 and f 2 will provide retarding torque, and hence angular retardation (say α ) about the center of the disc.
Writing torque about the center,
f 1 R + f 2 R = 2 m R 2 α
To find time taken to retard to 0 r a d / s ,
0 − ω 0 = − α t ⇒ t = α ω 0
Solving these equations, we get
f 1 = μ 2 + 1 μ 2 m g
f 2 = μ 2 + 1 μ m g
α = ( μ 2 + 1 ) R 2 ( μ 2 + μ ) g
t = 2 ( μ 2 + μ ) g ω 0 ( μ 2 + 1 ) R = 3