The great downhill race
4 objects A , B , C , D are made up of the same material. They are released, simultaneously from rest, from the starting line on the top of a rough inclined plane, so that they roll without slipping. A and B are cylinders, and C and D are spheres.
What is the order relation of the times taken by the objects to cross the finish line?
(A, B, C, D in the answer options denote the times taken by corresponding objects.)
Details and Assumptions:
-
The objects are released with all their centers above the same starting line, which is the top edge of the ramp. They are considered to have crossed the finish line--the red line near the bottom edge--only if their centers have crossed the line when viewed from the side.
-
Moment of inertia of a cylinder is 2 1 M R 2 and that of a sphere is 5 2 M R 2 , where M is the mass and R is the radius of the respective objects.
-
B has twice the radius of A , and D has twice the radius of C .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Good use of conservation of energy. It is interesting to see that the time it takes for the object to roll depends on neither the mass nor the radius of the object. It only depends on how the mass is distributed.
The time taken t is proportional to 1 + k and not k , but the relation still holds: greater the k , the more slowly it rolls
Log in to reply
I assumed that since k is a positive quantity, therefore accounting 1 + k and k under the proportionality sign would mean the same thing. Thank you, I'll make the corrections.
Log in to reply
We say that two variables are (directly) proportional to each other if their ratio is a constant. Here, the ratio of t and 1 + k is constant, so t is proportional to 1 + k . Ratio of t and k is not constant, so they are not directly proportional. Don't worry, it's not a big issue.
Does this take air resistance into account? (Not super farmiliar with the physics involved)
Log in to reply
At the speeds which the objects are moving, air resistance is negligible. The solution does not take into account air resistance. But it's an interesting question, because air resistance is dependent on the shape of the object, the surface area in contact and the velocity of the object. I'll go figure it out and post another version of this question, but underwater, where fluid resistance is not negligible:)
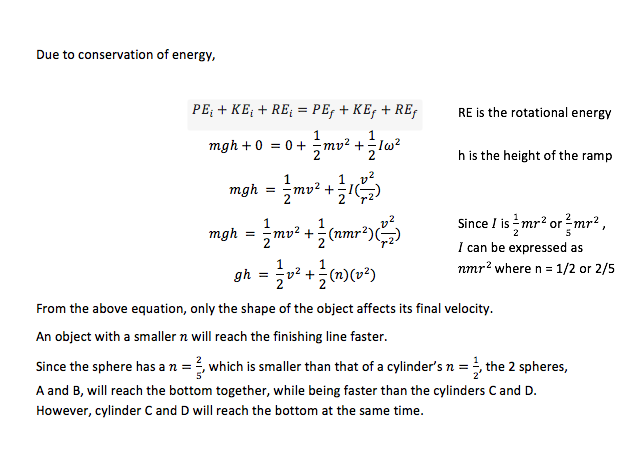
**Sorry, that's an error in the explanation. I meant to say that the spheres will reach the bottom first, followed by the cylinders. Ignore the last statement
Stating the final velocity is larger doesn't necessarily prove that it crosses the line first does it? Acceleration is what matters I believe.
Log in to reply
If the final velocity is greater then it must have a greater acceleration as well. I am using the equations, v 2 = u 2 + 2 a S . S and u are equal, therefore, v depends on the acceleration alone.
Does it matter that the spheres have less contact area on the ramp than the cylinders ?- the ramp is a rough inclined plane...
Log in to reply
No, in general, the friction does not depend on the contact area.
Log in to reply
Is it right to say that friction does not slow the objects down AT ALL since friction is required for rolling without slipping? Then is it also true that as long as 2 objects are rolling without slipping, friction will not affect it's acceleration/final velocity regardless of how large the frictional force is?
Log in to reply
@Chua Shao Cong – No, that is not correct. For the force equations, gravitational pull down the incline - frictional force = Mass times the acceleration of the center of mass. Thus, greater is the friction, lesser is the acceleration of the center of mass of the objects.
Log in to reply
@Rohit Gupta – I'm referring to ROTATING OBJECTS, not sliding objects. Friction is causing the rotation in the first place, and it's static friction. So it shouldn't matter if the friction is greater in this case right?
Log in to reply
@Chua Shao Cong – No, Newton's laws act on all the objects irrespective of them being in translatory motion or rotatory motion. F net = M a cm Thus, the friction will decrease the acceleration of the center of mass of the objects.
Log in to reply
@Rohit Gupta – But the friction in this case is static friction, and it is the force causing a torque on the objects, which causes them to rotate. Without friction, the object would simply slide down the ramp. And since it is not sliding, there should be no kinetic friction shouldn't slow it down. At least that's the hypothesis.
What I am asking is that does it matter if one object has a larger coefficient of friction than the other, for example, would a metal wheel roll faster/slower compared to a rubber wheel of the same size and mass?
Log in to reply
@Chua Shao Cong – First, even static friction can slow down the objects. Yes, it doesn't produce heat, but like you said it do create the rotatory motion. It takes the energy of the translatory motion and converts it into the rotatory form.
Secondly, the static friction does not depend on the coefficient of friction. So, both the metal wheel and rubber wheel will roll with equal speeds.
Walter Lewin demonstrates moment of inertia: https://www.youtube.com/watch?v=cB8GNQuyMPc
Log in to reply
Watch his explanation from 12:06, it gives you a different perspective to it's rotational kinetic energy
Whether the finish line crossing is viewed from the horizontal or perpendicular to the rolling surface, the larger objects have a greater vertical distance to descend, given that their centers of gravity are higher above the starting edge than the smaller objects.
Log in to reply
Won't their center of gravity be higher at the finishing line as well?
Log in to reply
The problem says the finish is the object's center crossing the line.
Apologies for the confusion, the question meant to ask "Which object attains the greatest final velocity after THEIR CENTER OF MASSES have travelled the same distance down the ramp"
Apologies for the confusion, the question meant to ask "Which object attains the greatest final velocity after traveling the same distance down the ramp"
I have not totally understood. I thought that each mass center feels the same component of the gravity acceleration, in particular the component parallel to the oblique plane. So their motion is supposed to be defined by the same cost. acceleration. Why shouldn't they cross the line at the same time?
Thanks
Log in to reply
They feel the equal gravity but different friction. The friction here will be static and hence, different objects will require a different friction to roll.
I think a better solution is that the transnational acceleration, a t, differs only by the constant n. a t = (sin(theta)*g)/(n+1).
Log in to reply
I suppose that would be a more intuitive answer. However, since the objects are traveling across the same distance down the ramp, you could use the equation v^2 = u^2 + 2as to figure out which one has the greatest acceleration either. But you do have a good point there. Perhaps you could suggest an alternative solution using dynamics instead?
Hey, can I ask how you managed to derive acceleration? I can't do it that without using the parallel axis theorem. I had to assumed the surface in contact to be the pivot, and used the parallel axis theorem to calculate the "new" moment of inertia.
Log in to reply
Yes, I used the contact point as the pivot point and the parallel axis theorem to derive the moment of inertia.
C and D are the spheres and A and B are the cylinders (according to the problem statement), have you just confused these in your explanation, or is there something wrong with your calculations?
Log in to reply
Sorry, that's an error in the explanation. I meant to say that the spheres will reach the bottom first, followed by the cylinders
The speed of rolling will differ if the spheres or cyclinders are hollow rather than solid. The problem does not state whether the objecgts are hollow or solid. Alan Feingold
Log in to reply
The problem stated the moment of inertia of the spheres and the cylinders. A hollow sphere or a cylinder would have a different moment of inertia.
I would assume the objects as solids unless and until stated otherwise.
What if this is a free fall with the edge angle of 90 degrees? Does that mean all the objects touch the ground at the same time?
Log in to reply
For free fall rotation is not factored in. All objects will be treated as a point mass and hence, they will touch the ground at the same time
If they start with their bottoms in one line, then their bottoms will hit the ground at the same instant, assuming the air drag to be zero.
Instead of energy if we just look from force perspective, the net acceleration of any object here is samr as given by,
a = gsin(e) - (m)gcos(e) Where (m) is coefficient of friction g is acceleration due to gravity (e) is angle of inclination
Given that all centre of mass travel same inclined distance shouldn't linear velocities be same finally? What am I missing in this analysis? Any other force acting here?
Log in to reply
The objects are rolling without slipping, won't the friction be static in nature?
The velocities will be the same only if the objects are not rotating. If you treat them as a point mass, then yes, you can use the equation mgh=1/2 mv^2. But if you're dealing with rotating objects, the kinetic energy is split between translation and rotation, which is what is shown in the solution
Log in to reply
I know the energy conservation. My doubt is how to solve the same question using force conversation.
Log in to reply
@Mac A – There will be three equations to solve the problem,
1) Force equation: F n e t = M a c m
2) Torque equation: τ c m = I c m α
3) Equation of rolling motion: a c m = R α
@Mac A – I can't manage to get LaTeX working for my comment, so here's a photo to my solution: https://goo.gl/JATZey
First you take the point where the object comes in contact with the ramp as the new pivot, then you find the new moment of inertia using the parallel axis theorem. Basically, you are treating the object as if it is rotating around the point of contact, instead of its center of mass.
As the objects will roll down without slipping on the inclined plane, assuming any particular body with radius r , mass m and radius of gyration equal to r k and using the principle of conservation of mechanical energy (with reference for potential set at the base of the plane) we get
m g h = 2 m v 2 + 2 I ω 2
As the motion is a pure rolling motion we have : ω = r v and so
⟹ ⟹ m g h = 2 m v 2 + 2 ( k m r 2 ) ( r 2 v 2 ) g h = 2 v 2 + 2 k v 2 v = 1 + k 2 g h
Now with the help of the equation : v 2 = u 2 + 2 a s , since u and s remain the same for all bodies, therefore a ∝ v 2 which also means that a ∝ ∣ v ∣ and the greater the acceleration, the less time it takes to cover the required distance s .
Thus we have
t ∝ a 1 ∝ v 1 ∝ 1 + k
So the spheres will take less time to reach the bottom as their 1 + k values are less than that of the discs. Also further note that the order is irrespective of the size of the body and only depends on its shape.
Thus, the correct order is : C = D < A = B .