The Inelastic Chain
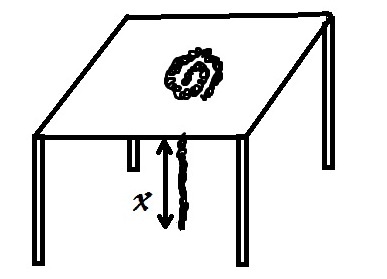 A chain of mass per unit length
is piled on top of a table. A small hole is cut, through which the chain starts falling. Assume that the only moving part of the rope is that which hangs through the hole, beneath the table. The section of rope on the table is at rest.
A chain of mass per unit length
is piled on top of a table. A small hole is cut, through which the chain starts falling. Assume that the only moving part of the rope is that which hangs through the hole, beneath the table. The section of rope on the table is at rest.
Find the velocity of the chain at seconds.
Details and Assumptions
The answer is 6.533.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the length of the falling chain be x at any time. The tension at the top end will be zero as the chain above it is loose. So, the only force acting on the falling chain is the gravitational force F = λ x g . Now we can't write F = m a as the mass is a variable, so we write:
F = λ x g = d t d p = d t d ( λ x v )
⟹ x g d t = d ( x v )
⟹ x 2 g ( v d t ) = x v d ( x v )
⟹ x 2 g d x = x v d ( x v )
On integrating this with the boundary condition that v = 0 when x = 0 , we get v = 3 2 g x … 1
⟹ d t d x = 3 2 g x
Solving this equation with the boundary condition that x = 0 when t = 0 , we get: x = 6 g t 2 .
Plugging this in equation 1 , we get:
v = 3 g t
Hence, the answer is v ( 2 ) = 6 . 5 3 3 .