The price of a pen

Relevant wiki: Floor and Ceiling Functions - Problem Solving

$11 < 9p < 12 \Rightarrow 1.222 < p < 1.333$

$15 < 13p < 16 \Rightarrow 1.153 < p < 1.231$

Now, it is easy to see (and even easier, if we put these two intervals above a number line), that the only solution with integer number of cents is:

$\boxed { \$ \text {1.23} }$

The answer can be found this way: (((15/13 +16/13)/2)+(11/9+12/9)/2))/2

Relevant wiki: Simple Equations

Obviously the price of the pen is $1 and some cents. Let x be the number of cents. Then 9x is larger or equal 200 but less than 300. On another hand, 13x is larger or equal 200 but less than 300. So x must be larger or equal 16 but less or equal 23. Thus x=23 cents. The price of the pen is $1.23.

$\text{suppose,pen=P}$

now, $11<9P<12$

or, $\frac{11}{9}$ < $\frac{9P}{9}$ < $\frac{12}{9}$

or, $1.22<P<1.33$ ..............(1)

again, $15<13P<16$

or, $\frac{15}{13}$ < $\frac{13P}{13}$ < $\frac{16}{13}$

or, $1.153<P<1.231$ ........(2)

from 1 and 2 ,we get, ................................................... $1.22<P<1.231$ . $\text{so, P must be=1.23}$ = $1.23 \times 100=123 cents$

I asked myself how could this still be intuitive for students of lower grades & I felt that the following approach (slightly brute force) might work.

Find the highest multiple of 13 between 1500 & 1600. (Review question: Why highest?) 1599

If the price of 13 pens is 1599, then what is the price per pen? 123 cents

If the price per pen is 123 what is the price of 9 pens? 1107

Is 1107 between 1100 & 1200? Yes

If not, let's find the next highest multiple of 13 between 1500 & 1600 & repeat.

Once they solve this, I think there is an opportunity to optimise, extract patterns & perhaps, arrive at a representative derivation of the answer. I thought this might help educators who are wondering how to use problems like these in class. Is there a simpler way? Would love to hear.

$9 \times 13 = 117$ pencils cost between $143 and $144, or between 14300 and 14400 cents. The only number in that region which is divisible by 117, is $14391 = 117 \times 123$

cost of 9 pens is b/w $11 & $12
so cost of 1 pen may be dollar $.....1.23, 1.32, 1.41, 1.50, 1.59, 1.68, 1.77, 1.86, 1.95,
$1.23 coincide only with ans. $1.23

Here is my solution. It may not be the optimal but it gets the job done.

arrayA = [] arrayB = []

p = 9 cMin = c = 1100 cMax = 1200

while (c <= cMax): r = c % p if(r == 0): m = c / p arrayA.append(m) c += 1

p = 13 cMin = c = 1500 cMax = 1600

while (c <= cMax): r = c % p if(r == 0): m = c / p arrayB.append(m) c += 1

for a in arrayA: for b in arrayB: if(a == b): print (a)

11/9+12/9+15/13+16/13= 578/117

Divide that by 4 (Average it)

You get 289/234

Which is equal to 1.235...

DONT FORGET!!!

It said how many CENTS is it,

It is 123 cents

The lowest value of x is defined by 9x=11, highest by13x=16. Thus 9x +13x=11=16 ie 22x=27 Therefore x=1.22777 which rounded to the nearest whole number of cents equals 123

Lets call the price $p$ . Then we have two inequalities $1100 \leq 9 p \leq 1200$ (1) and $1500 \leq 13 p \leq 1600$ (2). Now the folowing solution is relied to the beaty of 9 because of the fact that for every number multiple of 9 the sum of its digits equals 9 . For example $9\times 12 =108$ and $1+0+8=9$ . So inspired by inequality (1) we define $9 p =1100+x$ and also from this inequality we know that $x\leq 100$ . And cause of the fact that $1100+x$ must have sum of digits 9 we know that x is a one or two digit number with sum of digits $9-2 =7$ . From the second inequality we see that $13 (1100 + x)/9 \leq 1600 \Rightarrow x\leq 1600 \cdot 9/3 -1100 = 7.6...$ . So x is one digit number from 1 to 7 with "sum of digits" 7 so its 7 itself! From this we know that $p=(1100+7)/9=123$ . Notice the power of 9, the solution did not even need the left side of the inequality (2).

OHHH I DID ITTTTTTTTTTTT!!!!!!!!!!!!

Let cost in dollars be x given, 11<=9x<=12 and 15<=13x<=16 =>1.22<=x<=1.33 and 1.15<=x<=1.23 So, most possible value is 1.23

Solved with the following 3 lines of Python code:

• first = [i//9 for i in range(1100,1200) if i%9==0]
• second = [i//13 for i in range(1500,1600) if i%13==0]
• print(set(first).intersection(second))

Output: 123

Here was how I did it.

Review what we know

• We are told that if purchase 9 pens, the total price will be between $11 and $12.
• We are told that if we purchase 13 pens, the total price will be between $15 and $16.
• We are asked to report the cost of a single pen, in whole cents, so we can convert the dollar values to cents.

Write these as inequalities and using cents:

• The first is $1100 \le 9 pens \le 1200$
• The second is $1500 \le 13 pens \le 1600$

But we don't want to know the number for 9 or 13 pens, so let's divide both sides of the each inequalities to get the price for a single pen within that inequality. This also puts each inequality in comparable ranges.

• $(1100\div9)\ \le ((9 pens) \div 9) \le (1200\div9)$ which simplifies to $\approx122.2 \le 1 pen \le \approx133.3$
• $(1500\div13)\ \le ((13 pens) \div 13) \le (1600\div13)$ which simplifies to $\approx115.4 \le 1 pen \le \approx123.1$

From this we can figure out the most restrictive price for a single pen by taking the narrowest set of prices that are compatible with both inequalities.

• $\approx122.2 \le 1 pen \le \approx123.1$

This is because we know that the minimum cost for a pen has to be greatest of the possible minimums and the least of the possible maximums since that is the only way both inequalities can be true. We have combined the inequalities in the only manner which allows both original inequalities to be true. Therefore the cost of a single pen has to be between 122.2 and 123.1 cents. The only whole number of cents that falls between those two values is 123 cents.

Simplest way how not to do this: We have:

1 and 2 are situations. Worst and Best are cases. (Worst is expensive, Best is cheap). Now:

Step 1. Calculate the worst & best cases. B1= $\frac{11}{9}$ =1,22 ; W2= $\frac{12}{9}$ =1,33 ; B3= $\frac{15}{13}$ =1,15; W4= $\frac{16}{13}$ =1,23

Step 2. Calculate the worst & best equal cases. Beq= $\frac{11+15}{9+13}$ =1,18; Weq= $\frac{12+16}{9+13}$ =1,27;

Step 3. Find average. B1+W2+B3+W4+Beq+Weq / 6(count) = 1,23

Convert. 123 Cents.

Max price=15.99/13=1.23 Min price=11.01/9=1.22 Mean price=(1.23+1.22)/2=1.225 Integer price=123

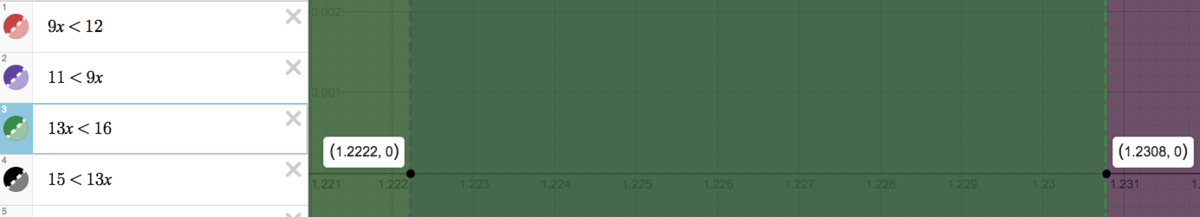Using this graph: https://www.desmos.com/calculator/uuhho50ch9 I could see that the value was between $1.2222 and $1.2308, so the answer in integer number of cents must be 123.

11 divide by 9 =1.22. 12 divide by 9 = 1.33 average 1.27 15 divide by 13= 1.15 16 divide by 13 = 1.23 average 1.19 Average of 1.27 plus 1.19 = 1.23 Price of one 123

Out of the two sets of pens, the one that requires the highest degree of accuracy is the set that has more pens. So if you calculate the maximum cost of each pen, then round down to the nearest whole number, you get your answer.

So, 16 dollars for 13 pens = (16/13) = 1.2307 = 1.23

The intuitive way. You can take max price for 9 pencils and it's $12. Then min price for 13 pencils which is $15. Then sum of pencils = 22 and all for $27. This gives you $1.227. Round it to nearest hundreds and convert to cents and it's 123.

Clearly 4 pens costs more than $4, and at most $5. If 4 pens cost $5, they'd cost $1.25 each so 9 would cost $11.25 and 13 would cost $16.25. We need to subtract an integer number of cents X from $1.25 such that 9X is less than or equal to 25 cents (so that 9 pens still cost at least $11), and such that 13X is greater than or equal to 25 cents (so that 13 pens now cost at most $16). 2 is the only such integer. So we subtract 2 cents from the $1.25 and we get that one pen costs $1.23.