The Shanghai Tower Is The Tallest In China. But The Chinese Are Flocking To It For Another Reason

Take a momment to think about the 'jerk' and how it works (in both ways). The solution to this problem is reached by assuming that the elevator goes from acceleration zero to $1.25$ in $0.5 sec$ then keeps a constant acceleration of $1.25$ for $14.15 sec$ to reach it's top speed of $18 m/s$ , then stays at top speed until it's time to decelerate... this methode spits out the answer $45.8 sec$ , but i think this is wrong, here is why :

After the period of constant acceleration we can't directly jump to zero acceleration once we hit the limit speed because then we wouldn't be obeing the 'jerk', so insead we should give time for the system to decrease it's acceleration (not decelarate! here we are still speeding up) from $1.25m/s^{2}$ to $0m/s^{2}$ . That would give us, from the start, a $0.5 sec$ time to reach a distance of $0.15625 m$ with a speed of $0.3125m/s$ , then a periode of $13.9 sec$ of constant acceleration in wich we travel $120.75625 m$ and reach the speed of $17.6875m/s$ , then a period of $0.5 sec$ of negative 'jerk' that will allow the acceleration to go from $1.25m/s^{2}$ to $0m/s^{2}$ while in accord with the limitations, and the speed to hit its limit at $18 m/s$ . After this we should account for the periode of constant speed, and finally mirror the first $3$ steps for the deceleration phase. This would yeld a final answer of $47.3 sec$ .

The most efficient motion for the elevator is to use maximum jerk for some period of time (say, $a$ seconds) to get up to maximum acceleration. Then, maintain this maximum acceleration for some period of time (say $b$ seconds) until the elevator is almost at maximum speed, and then use maximum negative jerk to reduce to zero acceleration as the elevator hits top speed. Then, maintain this top speed for some period of time (say $c$ seconds) until the elevator is approaching the top, at which time repeat the process in reverse.

This suggests that the jerk function will be a piecewise constant function. $J(x) = \left\{ \begin{array}{rcrcl} 2.5, & \text{for } & 0 \leq& x &< a \\ 0, & \text{for } & a \leq& x &< a + b \\ -2.5, & \text{for } & a + b \leq& x &< 2a + b \\ 0, & \text{for } & 2a + b \leq& x &< 2a + b + c \\ -2.5, & \text{for } & 2a + b + c \leq& x &< 3a + b + c \\ 0, & \text{for } & 3a + b + c \leq& x &< 3a + 2b + c \\ 2.5, & \text{for } & 3a + 2b + c \leq& x &< 4a + 2b + c \\ 0, &\text{otherwise} \end{array}\right.$ where $a, b, c$ are positive constants to be determined. The limitations on the elevator can be expressed as the following system of equations: $\begin{aligned} A(x) &= \int J(x) \mathrm{dx} \\ V(x) &= \int A(x) \mathrm{dx} = \iint J(x) \mathrm{dx} \\ D(x) &= \int V(x) \mathrm{dx} = \iiint J(x) \mathrm{dx} \\ |A(x)| &\leq 1.25 \\ |V(x)| &\leq 18 \\ |D(x)| &\leq 557 \end{aligned}$ This is a straightforward, if very tedious, system of equations to solve, with 3 equations in 3 unknowns. Doing this by hand is arduous, but a CAS or similar can do this easily, yielding the required solution.

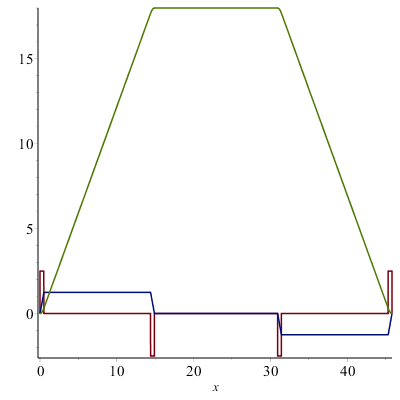
Here's a plot of the jerk (red), acceleration (blue), and velocity (green). Note that the velocity is actually continuously differentiable and has subtle curves instead of sharp edges. Here's a plot of the elevator's displacement. 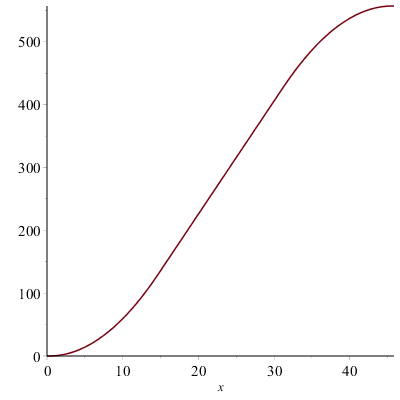The answer to the problem is simply the value of $x$ for which the maximum displacement is achieved, which is $\frac{2063}{45} \approx \boxed{45.8}$ .

It ll take 0.5 seconds to reach the top acceleration of 1.25. Use integral calculus to find formula for s in jerk situation ( function of time cubed). It takes another 14.15 seconds to reach the top speed of 18. Overall this process has to replicated while stopping. This will give us the time taken in this process as well as distance covered. The rest of the distance is covered at a steady speed of 18.