The Sunset

Hi @Justin Travers ! Your question is pretty interesting, so I tried to "model" the refraction of the atmosphere with simple model, to give you an idea of how we could consider that.

Let's consider an atmosphere with radius $R_E + h$ and a single refraction coefficient $n_A$ . In reality, the coefficient depends on the height of the atmosphere, so we should integrate the influence of it over the height (this would complicate the problem a lot).

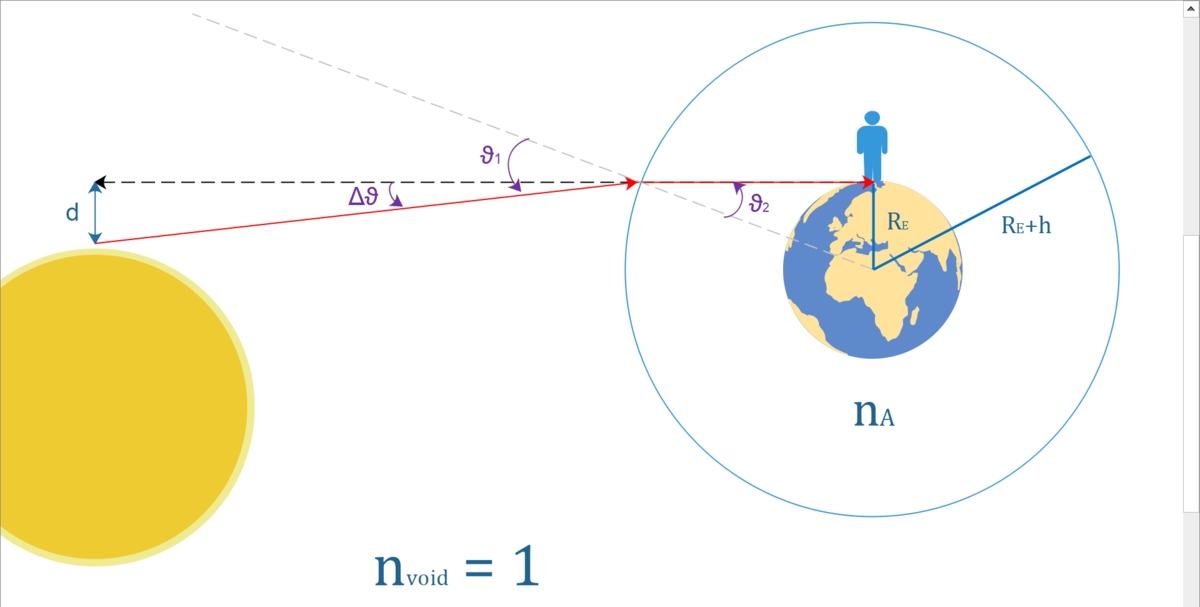
Now that we have a model and a drawing, we can use Snell's Law (https://brilliant.org/wiki/snells-law/) to relate the angle of incidence $\theta_1 = \theta_2 +\Delta \theta$ and the angle of refraction $\theta 2$ :

$\boxed{ n_{void} \sin{\theta_1} = n_{A} \sin{\theta_2} }$

( $n_{void} = 1$ )

Using some geometry, we can determine the sine of the angle of refraction: $\sin{\theta_2} = \frac{R_E}{R_E+h}$ . The expression simplifies to:

$\theta_1 = \sin^{-1} \left[ n_{A} \frac{R_E}{R_E+h} \right]$

...so the angle $\boxed{ \Delta \theta = \sin^{-1} \left[ n_{A} \frac{R_E}{R_E+h} \right] - \sin^{-1}\left[\frac{R_E}{R_E+h}\right] }$

With this angle, we can now determine the distance of the sun under the horizon $d$ :

$d \approx (D_{ES}-h)\cdot \Delta \theta \approx D_{ES} \cdot \Delta \theta$ .

So back to the solution of the original problem, during the measured time $\Delta t$ the sun travelled $2R_S + d$ :

$2\pi \frac{\Delta t}{\tau} = \frac{2R_S + D_{ES} \Delta \theta}{D_{ES}} \Longrightarrow \frac{R_E}{D_{ES}} = \pi \frac{\Delta t}{\tau} - \frac{\Delta \theta}{D_{ES}}$

and we get then finally for the temperature of the sun:

$\boxed{ T_S = \frac{T_E}{\sqrt{ \frac{\pi}{2} \frac{\Delta t}{\tau} - \frac{1}{4} \left( \sin^{-1} \left[ n_{A} \frac{R_E}{R_E+h} \right] - \sin^{-1}\left[\frac{R_E}{R_E+h}\right] \right) } } }$

I want to say it again: this is an oversimplified model, but is a good way to maybe get an estimation. If we only consider the Troposphere, then $n_A \approx 1.0003$ and $R_E + h \approx 6370 + 12 \text{km}$ . Considering this, we get $\Delta \theta = 5 10^{-3} \text{rad}$ and $T_S = 8192 \text{K}$ .

Wow! This result surprised me. I am not a physicist, so I didn't expected the influence of refraction to be that big. For this problem I calculated a $\Delta t$ to get exactly $T_S = 5778 \text{K}$ as a result. I think that a real sunset lasts more than 2 min and 17 s.

I added as an assumption to the problem that the refraction has to be neglected, even if in reality it has a big influence. Considering refraction would make this problem extremly hard ;)

If there is an error in my argumentation, I am interested in your opinions!

@Justin Travers , take a look at my new problem, "the Sunrise". This problem might sound familiar to you ;)

Hi @raj abhinav , Both distances are almost the same. With $D_{ES}$ I meant "Distance earth-sun". The radius of the Earth is neglectable compared to the distance between both bodies, so the distance earth-sun ist almost equal to the distance sun-'earth's pole'. You can check that with some pythagorean geometry:

Where $x$ is equal to the distance from the pole to the sun. Pythagoras tells us that: $x^2 = D_{ES}^2 + R_E^2$ . But $R_E = 6,371 \cdot 10^3 \text{km}$ while $D_{ES} = 149,60 \cdot 10^6 \text{km}$ So $x^2 \approx D_{ES}^2$ .

I hope this answers your question! If I missunderstood you, let me know.