Still a Messy Close Form?
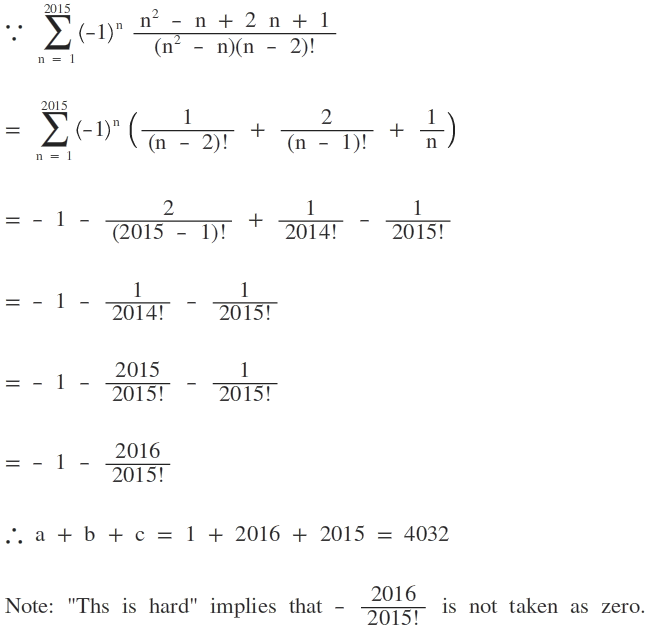
n = 1 ∑ 2 0 1 5 [ ( − 1 ) n ( n ! n 2 + n + 1 ) ]
If the summation equals to − a − c ! b for positive integers a , b , c
What is the minimum value of a + b + c ?
The answer is 4032.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Math man toogle the latex and copy the latex and edit your question because the 1 you have written looks like 2
Log in to reply
OK , it is 4032:)
Log in to reply
thanks for the help , please do it quickly so others don't confuse and from toogling other's people latex you can learn to write in latex
My break is more numerical for numerical analysis. Not as good as f (n) + f (n + 1).
Did exact same!!! Gd solution...
How to do it the other way
notice that if we switch 2015 to 1,3,5,7.... we see
when 2015 is 1 it is − 1 − 1 ! 2
when 2015 is 3 it is − 1 − 3 ! 4
.....
thus when 2015 is 2015 it is − 1 − 2 0 1 5 ! 2 0 1 6 )
so, a=1,b=2016,c=2015,a+b+c= 4032
So in a way you are doing mathematical induction . Good
for writing in fraction use - \dfrac{2}{1!} when encased in brackets , it will give - 1 ! 2
Log in to reply
Log in to reply
toogle latex is made so that one can learn latex. thanks
/dfrac{2}{5}
n = 1 ∑ 2 0 1 5 ( − 1 ) n ( n ! n 2 + n ! n + 1 )
n = 1 ∑ 2 0 1 5 ( − 1 ) n ( ( n − 1 ) ! n + n ! n + 1 )
− 1 − 1 ! 2 + 1 ! 2 + 2 ! 3 − 2 ! 3 − 3 ! 4 + . . . ( − 1 ) 2 0 1 5 ( 2 0 1 4 ! 2 0 1 5 + 2 0 1 5 ! 2 0 1 6 )
We can see that alternate terms cancel each other
− 1 − 2 0 1 5 ! 2 0 1 6
a + b + c = 4 0 3 2