To The End of The Line

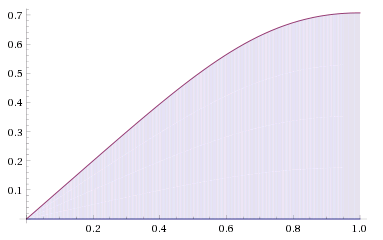
Rewrite the limit/sum as

$\lim_{n \rightarrow \infty} \left( \dfrac{1}{n} \displaystyle\sum_{k=1}^{n} \dfrac{\frac{k}{n}}{\sqrt{1 + (\frac{k}{n})^{4}}} \right).$

We can then see that this is a right-hand Riemann approximation of the integral

$\displaystyle\int_{0}^{1} \dfrac{x}{\sqrt{1 + x^{4}}} dx.$

As the integrand is Riemann integrable, our limit will be equal to this definite integral.

To solve this integral let $x^{2} = \tan(\theta).$ Then $2x dx = \sec^{2}(\theta) d\theta,$ and thus the integral (without bounds) becomes

$\dfrac{1}{2} \displaystyle\int \dfrac{\sec^{2}(\theta)}{\sqrt{1 + \tan^{2}(\theta)}} d\theta = \dfrac{1}{2} \int \sec(\theta) d\theta = \dfrac{1}{2} \ln |\sec(\theta) + \tan(\theta)| =$

$\dfrac{1}{2} \ln |\sqrt{1 + x^{4}} + x^{2}|,$

which when evaluated from $x = 0$ to $x = 1$ yields a value of $\dfrac{1}{2}*\ln(\sqrt{2} + 1).$

Thus $a + b + c + d = 1 + 2 + 1 + 2 = \boxed{6}.$