Triangles Being Fair
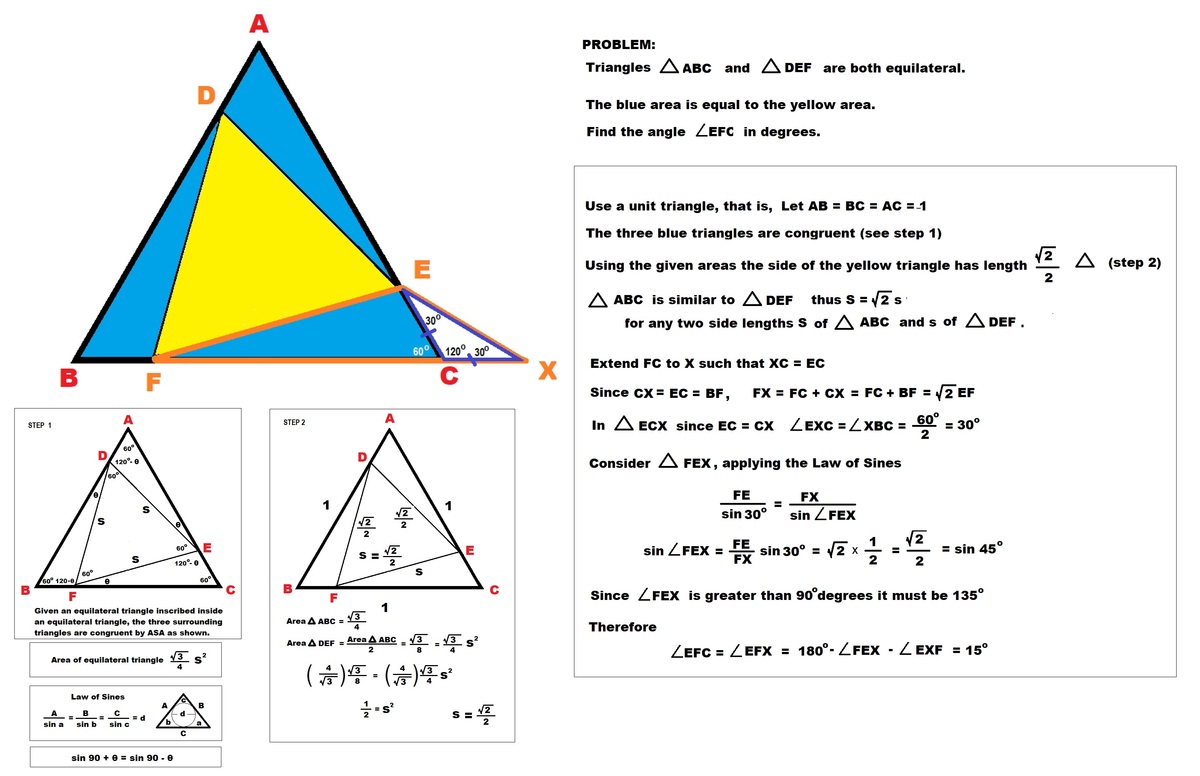
Triangles A C B and D E F are both equilateral, and the blue area is equal to the yellow area. Find the acute angle ∠ E F C in degrees.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
We can also apply cosine rule directly to calculate a :
a 2 + ( 1 − a ) 2 − 2 a ( 1 − a ) cos 6 0 ∘ = b 2 ⇒ 6 a 2 − 6 a + 1 = 0
And what if we do like this ?
Extend
F
C
to
X
such that
X
C
=
E
C
.
Then, we have
F
X
=
F
C
+
C
X
=
F
C
+
E
C
=
2
E
F
.
In triangle
E
C
X
, since
E
C
=
C
X
hence
∠
E
X
C
=
∠
X
E
C
=
2
6
0
∘
=
3
0
∘
.
Consider triangle
F
E
X
. Applying sine rule, we get
sin
3
0
∘
F
E
=
sin
F
E
X
F
X
⇒
sin
F
E
X
=
F
E
F
X
sin
3
0
∘
=
2
×
2
1
=
2
2
.
Since
∠
F
E
X
is greater than
9
0
∘
, hence it must be
1
3
5
∘
.
As such, we have ∠ E F X = 1 8 0 ∘ − ∠ F E X − ∠ E X F = 1 5 ∘ .
Log in to reply
I knew there had to be a geometry way, without trig. Thanks. (There is a typo, F E D = 1 3 5 ∘ .)
Log in to reply
It is still a trig solution. With the assistance of the nice geometric construction we get a very familiar value of sine and do our own inverse sine function "by inspection" instead explicitly invoking the arcsin then using tables or a calculator on the less familiar sine of 15 degrees. It still invokes the law of sines which is classed as trigonometry in most places.
I am also looking for a pure geometric solution, or at least one that does not depend on use of an inverse trig function to obtain the angle. I find this post incomprehensible as well as approval of it apparently without reading. In particular, DC and EC are given points in the original problem that cannot be "produced" to have lines of equal length. FED (in any order) is given to be 60 degrees in the diagram as a corner of an equilateral triangle and cannot be 135 degrees.
Log in to reply
Thanks. He made the mistake of letting his notation do double duty (in part because he wasn't focused on D in the diagram). I've edited it to point X instead.
Log in to reply
@Calvin Lin – Thank you for clearing that up. I have made a self-contained graphic from it and added a few things to make every step explicit and tacked it on to my first submission. (You may need screen magnifier to read it.) However, after digesting this, it seems only a little bit more "pure geometry" than than the proof above. Using geometry to get a very familiar value for sine is a still a sneaky use of an inverse sine operation, but by "inspection" rather than explicit invocation, then resorting to the tables or the calculator. It still invokes the Law of Sines, and that is trigonometry.
Log in to reply
@Robert DeLisle – I agree that this solution doesn't count as a purely synthetic approach. Thanks for adding in the details.
Log in to reply
@Calvin Lin – After going over all this in detail, it seems that the 30-60-90 approach is the least heavy duty in terms of trigonometry, thus closest to "pure geometry" after all . Arriving at the expression for the value of sine, and the equation for side lengths use only geometric side and area derivations based on the Pythagorean theorem. The bare definition of sine as ratio of sides and use of an inverse trig function are the only trig elements.
I was trying to find some way to get it from side/area arguments possibly with some construction into ordinary angle chasing somehow with no trace of trigonometry.
A solution based on a 30-60-90 triangle at a corner that connects the given areas to the side lengths and the angle. This approach limits the dependence on trigonometry to the definition of sine and use of the arcsin to obtain the angle.
Also a write up of a solution above that avoids the use of the arcsin function to get the angle. (However, arranging to the get a very familiar value of sine that we recognize allowing the reader to perform the arcsin function "by inspection" seems to amount to doing the same thing. One way or another it is still trigonometry at work here.)
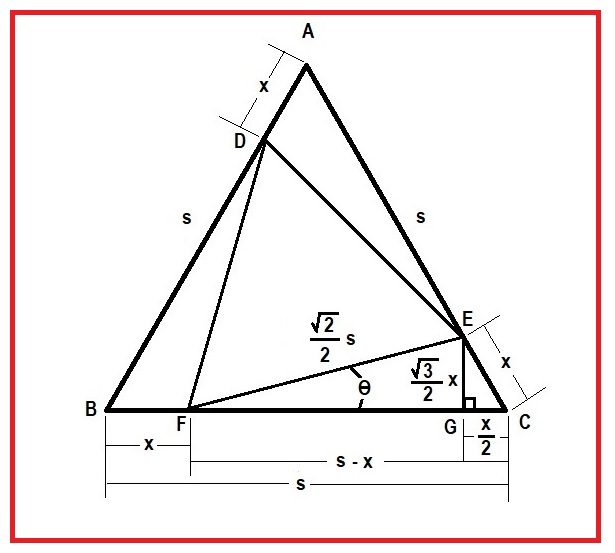
Let s be the side length of △ A C B , x be the shortest distance from a corner of △ A C B to a corner of △ D E F , and θ = ∠ E F C as shown in the diagram above.
From given information Area △ A C B = 4 3 s 2 , Area △ D E F = 2 1 Area △ A C B = 8 3 s 2 , Area △ E C F = 6 1 Area △ A C B = 2 4 3 s 2 and E F = 2 2 s .
Construct the perpendicular E G as shown to form a 30-60-90 triangle, △ E C G from which it follows that
(1) sin θ = 2 2 s 2 3 x = 2 3 s x = 2 6 s x and ∠ E F C = arcsin ( sin θ )
The value of x can be found using the side and area relationships as follows:
Area △ E C F = 2 4 3 s 2 = 2 1 s − x 2 3 x yields the equation 0 = x 2 − s x + 6 s 2
This equation has two roots a = 6 s ( 3 + 3 ) and b = 6 s ( 3 − 3 )
Using x = b in (1) gives the solution ∠ E F C = 1 5 ∘
Using x = a in (1) gives θ = 7 5 ∘ that is ∠ D F C corresponding to the other corner of △ D E F at D.
Note that the roots sum to 1. Both are the lengths of line segments on a side of the outer triangle.
so confusing
Put members of∆ ABC = m and k for ∆ EFD . area of blue zones = area of yellow zones so .5×m^2×√3\2 - .5×k^2×√3\2=.5×k×k×√3\2 so m=√2.k from geometry of the triangles ad=bf=ec=y as blue area =yellow so {√3÷4}.k^2=3×.5×y×(√2.k-y)×(√3÷2) so. k^2=3√2.ky-3y^2 ,, y^2-√2ky+(k^2÷3)=0. K Is constant so by solving the equation y=((√3-1)÷√6).k or( (√3+1)÷√6).k rejected as y must be less than k so y=.298858490k , at ∆ efc put angle (efc)=h cos(h)=k^2+(1.115507k)^2-(.298858490k)^2÷(2.k.(1.115507k)). So h=15°######
Define a = F C , and get E C = 1 − a .
Apply law of cosines to △ E F C to get an equation for a
b 2 = 2 1 = a 2 + ( 1 − a ) 2 − 2 a ( 1 − a ) cos 6 0 ∘
Simplify to 6 a 2 − 6 a + 1 = 0
And solve a = 2 1 + 6 3 or a = 2 1 − 6 3 .
If F C is the former, then E C will be the latter (and the smaller of the two).
Within △ E F C , angle x can be obtained from the law of sines 2 1 − 6 3 sin x = 2 1 sin 6 0 ∘
x = arcsin ( 2 2 3 − 1 ) = 1 5 ∘
(This solution was improved using Calvin Lin's suggestion, see comment below. My original solution compared the area of △ E F C calculated as a sixth of the area of △ A B C to the same area calculated using two sides and an angle to produce the equation for a .)