Try geometry

In the coordinate plane, let $A = (0,-11)$ , $B = (7,13)$ , and $P = (x,0)$ . Then $f(x) = AP + PB$ . The minimum occurs where $AB$ crosses the $x$ -axis. The equation of line $AB$ is $y = \frac{24}{7} x - 11$ . Setting this to 0 and solving, we get $x = \frac{77}{24}$ .

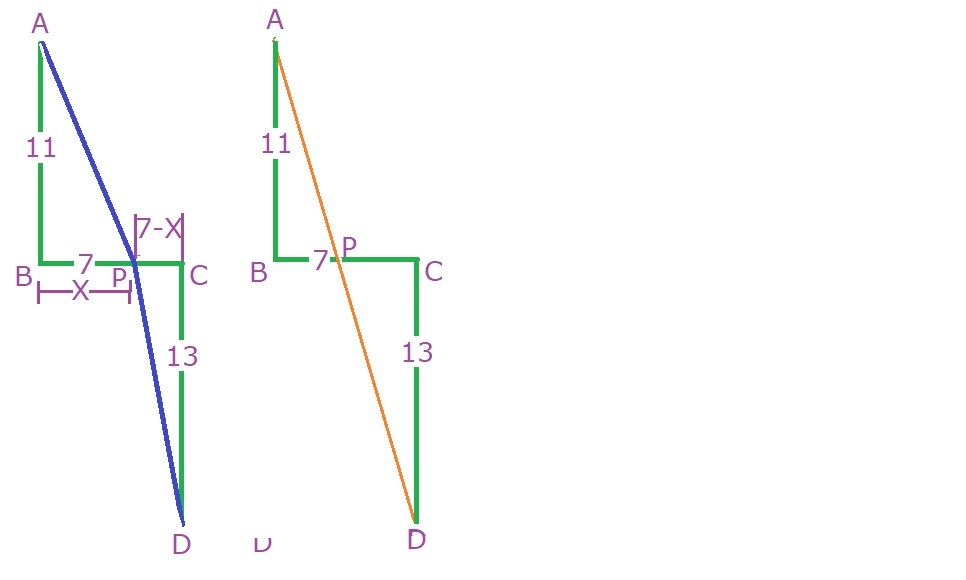
First, rewrite $f(x)$ as $f(x) = \sqrt{x^{2} + 11^{2}} + \sqrt{(x - 7)^{2} + 13^{2}}.$

Now draw a line $BC$ of length $7.$ Next, draw a line $AB$ of length $11$ extending upward from $B$ perpendicular to $BC,$ and a line $CD$ of length $13$ downward from $C$ perpendicular to $BC.$ Let $P$ be a point on $BC$ such that $|BP| = x,$ making $|PC| = 7 - x.$

Then since we will then have $|AP|^{2} = |AB|^{2} + |BP|^{2} = 11^{2} + x^{2}$ and $|PD|^{2} = |PC|^{2} + |CD|^{2} = (7 - x)^{2} + 13^{2},$ we see that $f(x) = |AP| + |PD|.$

Now by Fermat's principle we know that $f(x)$ will be minimized when $AD$ is a straight line, in which case $\Delta ABP$ and $\Delta DCP$ are similar. This in turn implies that

$\dfrac{|AB|}{|BP|} = \dfrac{|CD|}{|PC|} \Longrightarrow \dfrac{11}{x} = \dfrac{13}{7 - x} \Longrightarrow 77 - 11x = 13x \Longrightarrow x = \dfrac{77}{24}.$

Thus $a + b = 77 + 24 = \boxed{101}.$

Calculus approach

$\displaystyle f(x)=\sqrt{x^{2}+121}+\sqrt{x^{2}-14x+218} \implies f'(x)=\frac{x}{\sqrt{x^{2}+121}}+\frac{x-7}{\sqrt{x^{2}-14x+218}}$

$\displaystyle f(x_0)\leq f(x), \forall x \in \mathbb{R} \implies f'(x_0)=0$

$\displaystyle \frac{x_0}{\sqrt{x_0^{2}+121}}+\frac{x_0-7}{\sqrt{x_0^{2}-14x_0+218}}=0 \implies \sqrt{\frac{x_0^{2}-14x_0+218}{x_0^{2}+121}}=\frac{-(x_0-7)}{x_0}$

$\displaystyle \frac{x_0^{2}-14x_0+218}{x_0^{2}+121}=\left( \frac{x_0-7}{x_0} \right)^{2} \iff \frac{(x_0-7)^{2}+13^{2}}{x_0^{2}+11^{2}}=\frac{(x_0-7)^{2}}{x_0^{2}}\iff$

$\displaystyle \iff \frac{(x_0-7)^{2}+13^{2}}{(x_0-7)^{2}}=\frac{x_0^{2}+11^{2}}{x_0^{2}} \implies 1+\frac{13^{2}}{(x_0-7)^{2}}=1+\frac{11^{2}}{x_0^{2}}$

$\displaystyle \left[\frac{13}{(x_0-7)}\right]^{2}=\left( \frac{11}{x_0}\right)^{2} \implies \left| \frac{13}{(x_0-7)}\right|=\left| \frac{11}{x_0}\right| \implies \left|13x\right|=\left|11(x_0-7)\right|$

$\displaystyle x_0'=\frac{77}{24} \wedge x_0''=-\frac{77}{2} \implies f(x_0')=25 \wedge f(x_0'')\approx 87.36$

$\displaystyle \implies f(x_0')<f(x_0'') \implies x_0=x_0'=\frac{77}{24} \implies \boxed{a+b=77+24=101}$

Taking the "I dont wanna do complex calculus" approach, we can plug the equation into wolfram to find the minimum:
wolfram

it tells us that the global minimum is 25 when x= $\frac{77}{24}$
therefore; a is 77, b is 24, and 77+24 = 101

Desmos

Minimum occurs on the path of light travelling where reflection considering X axis as a mirror is a requisite!😍