Uniquity of an Equilateral
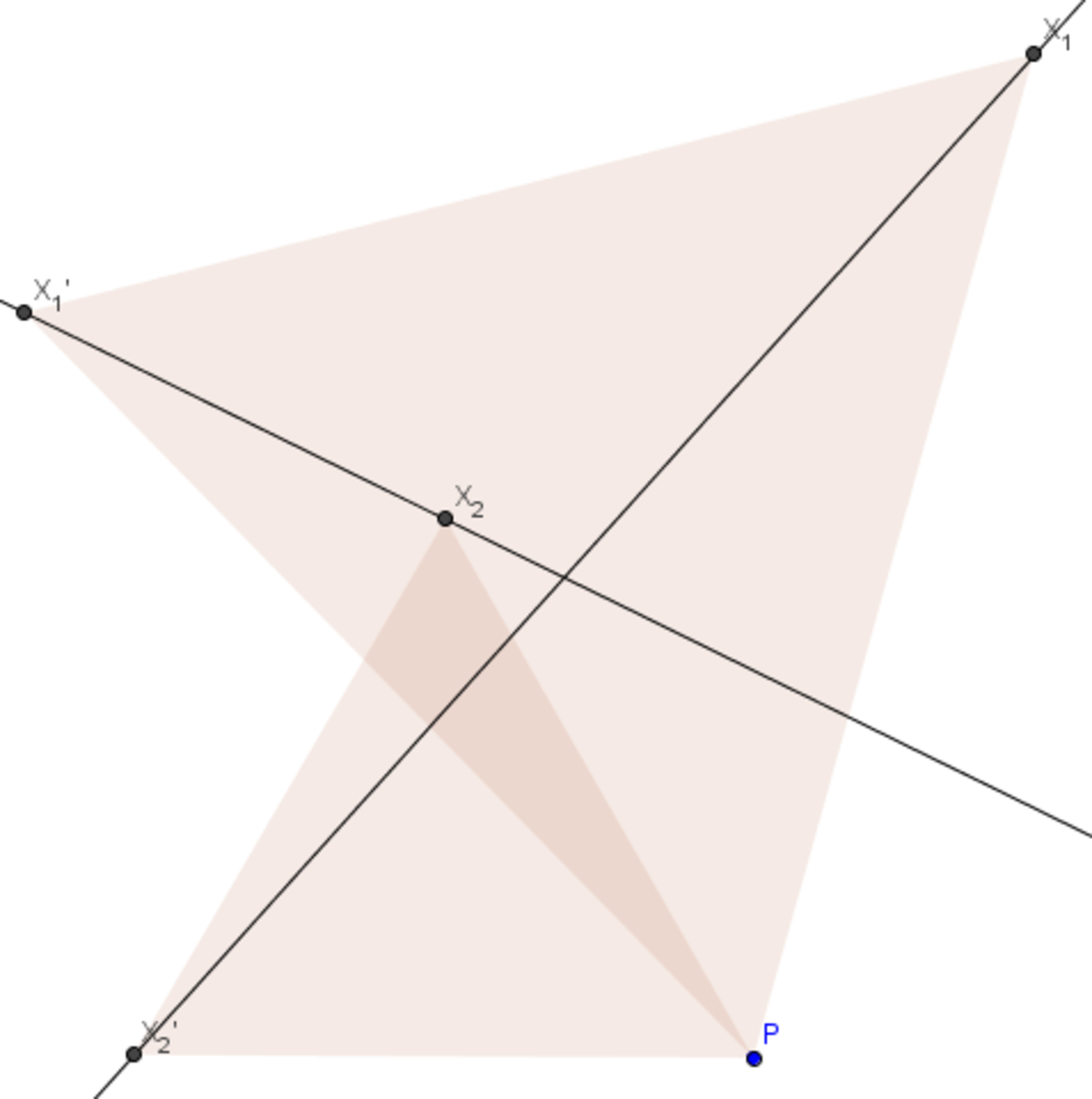Two lines
and
intersect at an angle of
. A point
is randomly chosen in the same plane as the two lines. If there is exactly one equilateral triangle with one vertex as
, one vertex on
, and one vertex on
, then find the sum of all possible values of
.
Details and Assumptions
The answer is 60.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
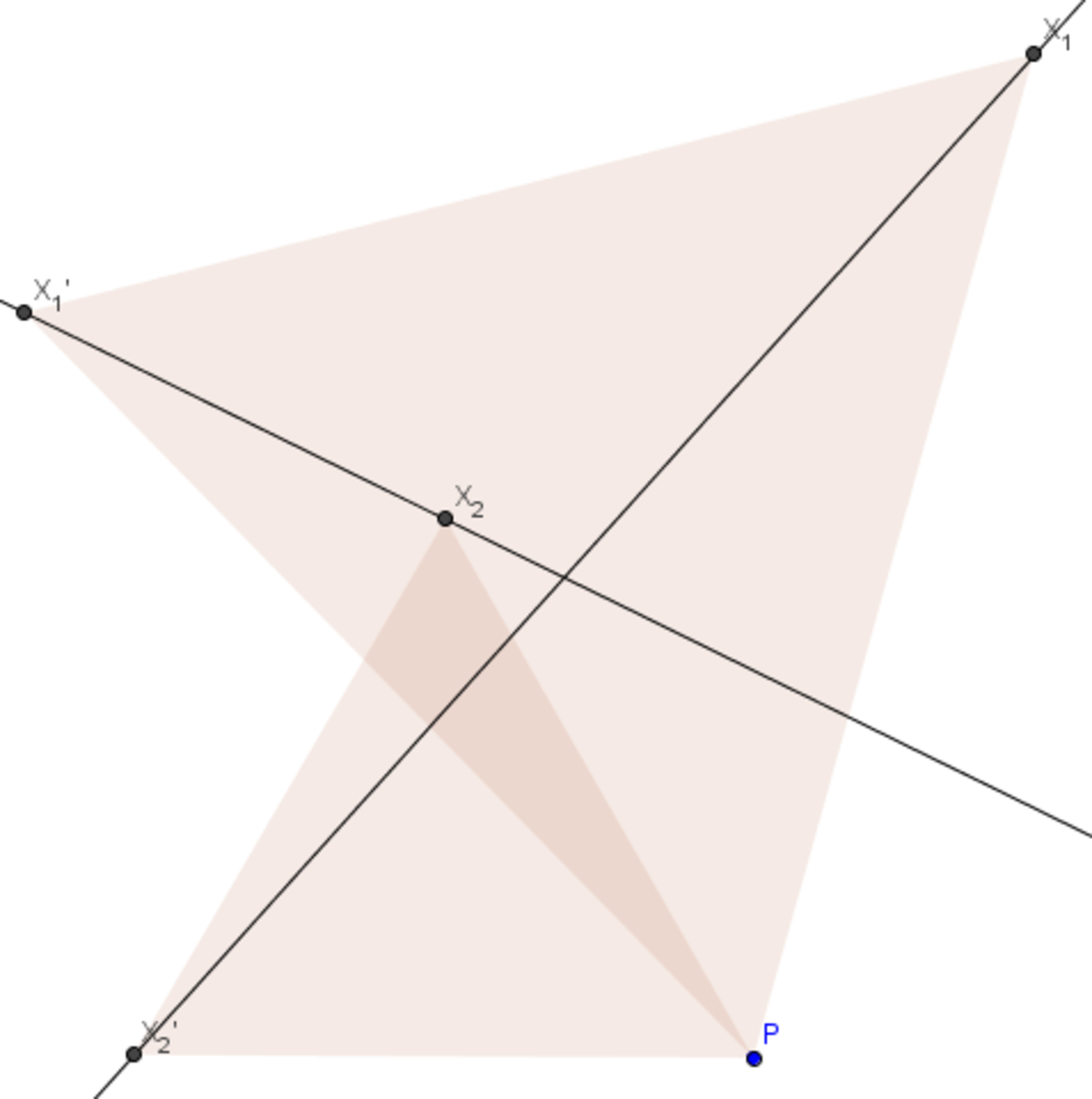
To have other two vertex on the two lines~~P must be symmetrically placed w.r.t. these two lines. So, it must be on the angle bisector. The angle at P must be 60. So that the other angles of the triangle be 60, the triangle with the point of intersection of the two lines also must be 60. In fact, the two triangles, are the two halves of a rhombus. ....>So the lines are at 60 degrees. The is no other solution.