LogCeption
 If the domain of the function
ln
(
ln
(
ln
(
ln
(
ln
(
x
)
)
)
)
)
is
(
a
,
∞
)
, solve for
a
.
If the domain of the function
ln
(
ln
(
ln
(
ln
(
ln
(
x
)
)
)
)
)
is
(
a
,
∞
)
, solve for
a
.
Note :
-
e is a mathematical constant which approximates to 2 . 7 1 8 2 8 .
-
We denote the tetration notation: n e = n number of times e e e . . . e
Image Credit: Meme Generator
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
@brian charlesworth Actually I didn't understand what you tried to point out before. Please can you explain ? Or just tell me where I had a mistake.
Thank you :)
Log in to reply
The question was worded quite differently before; it resulted in a domain error, since the answer would have required a calculation of ln ( 0 ) . You can check out the dispute section for a discussion on how the original question needed to be rephrased. There was a series of comments after Trevor's solution relating to this as well, but those seem to have disappeared, (at least for me).
Nice solution, by the way. :)
I just reverse-engineered it starting from the limiting case:
ln ( 0 ) = ln ( ln ( 1 ) ) = ln ( ln ( ln ( e ) ) ) = ln ( ln ( ln ( ln ( e e ) ) ) ) = ln ( ln ( ln ( ln ( ln ( e e e ) ) ) ) ) ⇒ a = e e e
Moderator note:
Great job! Can you generalize this for n ln ( x ) , where the logarithmic function is composites itself n times?
Nice, this is similar to my solution but yours is much easier to understand.
The smallest value of x in ln ( x ) is \color \green{0} .
Thus \ln(\ln(x)), \ln(x)=0\Longrightarrow x=e^{\color \green{0}}=\color \red{1}
\ln(\ln(\ln(x)))\Longrightarrow \ln(\ln(x))=0 \Longrightarrow \ln(x)=1 \Longrightarrow x=e^{\color \red{1}}=e
Notice the pattern: e to the power of the answer to the previous line (shown by the colors). When there are 3 natural logs. We have x = e . When there are 4, x = e e
Thus when there are 5. Our answer will be e^e^e
We can approach this value of x , but if we were to just plug in this value then there would be a domain error on the last ln calculation, since we would be attempting to calculate ln ( 0 ) . So technically, of the options provided, e e e e is the least number that will yield a real value for the given expression. There is in fact no smallest real value; if we let x = e e e + α for α > 0 then as we let α → 0 from the right then the given function will yield a more and more negative value, but as there is no "least value" for α > 0 there can be no least value for the given expression. So for this reason I chose e e e e .
Log in to reply
Hmm. You do have a point there, lol, I never thought of that. You really never miss a detail.
Could you report it please?
Log in to reply
Okie dokie; all done. I'm glad I had your blessing to dispute your question, otherwise I would never have done so. :)
Log in to reply
@Brian Charlesworth – Haha, I'm the same way. I tend to either talk it over with some of my friends on brilliant or with the problem creator before reporting it.
Log in to reply
@Trevor Arashiro – try replacing "is a real number" to "is a positive number"
@Trevor Arashiro – I've proposed a second remedy in the dispute section that just might do the trick. See what you think. :)
The notation ( α , ∞ ) refers to an open interval, which means that the terms on the left and right are not included in the interval
Log in to reply
Yes, I realize that. I was responding to a previous wording of the question, which would have led to a domain error, since it would have required the calculation of ln ( 0 ) , an impossibility. The present wording came at my suggestion, as documented in the dispute section to this question.
Ah dang, was fooled by the open parenthesis on ( a , ∞ )
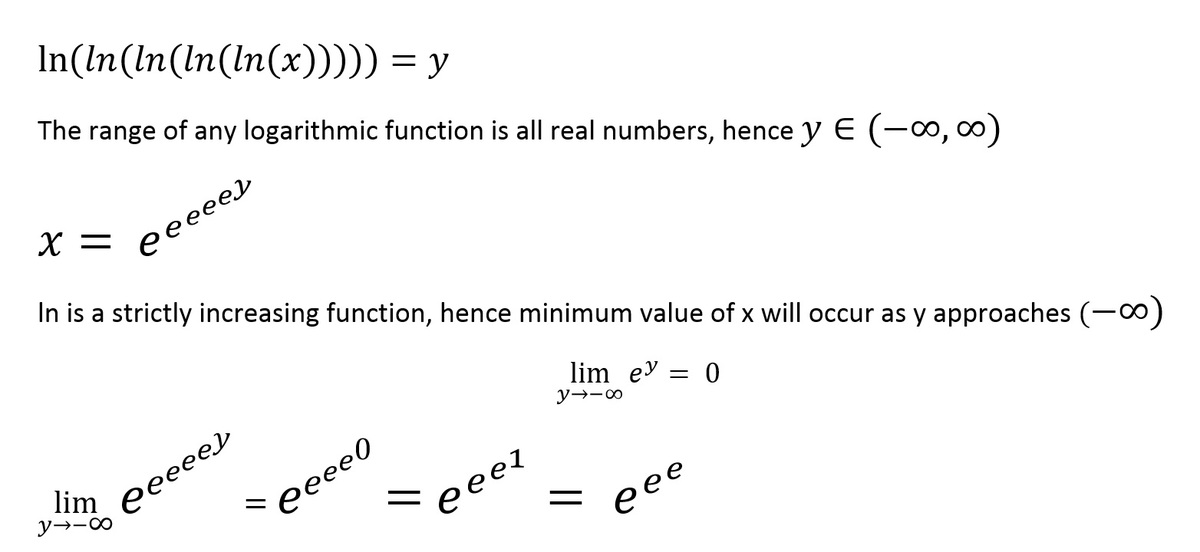
The argument of ln ( x ) should be positive :
= > ln ( ln ( ln ( ln ( x ) ) ) ) > 0
e x is strictly increasing :
= > e ln ( ln ( ln ( ln ( x ) ) ) ) > e 0
= > ln ( ln ( ln ( x ) ) ) > 1
Repeating the process:
= > e ln ( ln ( ln ( x ) ) ) > e 1
= > ln ( ln ( x ) ) > e
Again:
= > l n ( x ) > e e
= > x > e e e