What is distance CD?
This problem’s question: What is distance CD?
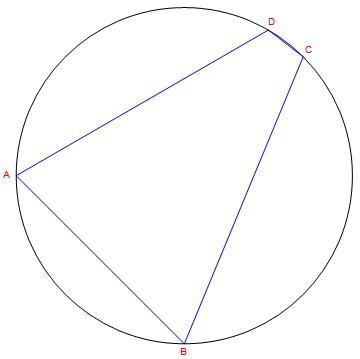
The points labeled A, B, C and D are on some circle. The center and radius are not provided.
AB AC AD BC BD = 2 = 2 + 2 = 3 = 2 + 2 = 3 + 2
The answer is 0.261052384440103.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
@Randolph Herber , we should use reference in Brilliant.org if it is available such as Ptolemy's theorem .
Prove - The points labeled A, B, C and D are on some circle?
That is a given fact in this problem. It is impossible to prove that the corners are on a circle with only 5 distances specified without, in effect, proving Ptolemy s Theorem in the process..
Log in to reply
It is actually possible to prove/disprove Yuriy's question.
We know that the sum of opposite angles of a cyclic quadrilateral is equal to 1 8 0 ∘ . Use the property cos θ = − cos ( 1 8 0 ∘ − θ ) and double check whether the cosine rule holds.
Log in to reply
No problem. The condition "The points labeled A, B, C and D are on some circle" is redundant.
From A B 2 = A D 2 + B D 2 − 2 A D ⋅ B D ⋅ cos ∠ A D B and A B 2 = A C 2 + B C 2 − 2 A C ⋅ B C ⋅ cos ∠ A C B we have ∠ A D B = ∠ A C B .The equivalent of this angles give - ABCD - is a cyclic quadrilateral.
2 = 6 − 3 3 + 2 + 3 1
What you are doing is proving Ptolemy's Theorem. I do not see that is comment adds anything to the solution.
Log in to reply
@A Former Brilliant Member – Yes, one way to prove Ptolemy's theorem is to apply Cosine rule.
I just want to point out that it is actually possible to prove that the corners are on a circle with the 5 distances specified.
Log in to reply
@Pi Han Goh – I say it again because you apparently did not get the point the first time: What you are doing is proving Ptolemy's Theorem. I do not see that that comment adds anything to the solution. Therefore, please stop sniping.
Log in to reply
@A Former Brilliant Member – Oh sorry, my reasoning was circular. I didn't realize my error until I scribbled more notes on my notepad.
Thanks for correcting my error.
Now I'm wondering whether we can still solve this question if one of the values is omitted.
See Ptolemy's theorem .
If a quadrilateral is inscribable in a circle then the product of the measures of its diagonals is equal to the sum of the products of the measures of the pairs of opposite sides.
In the following paragraph, cd is a two character variable name and not the product of c and d .
Solving 2 + 2 3 + 2 = 2 cd + 3 2 + 2 ] for the distance CD gives 2 − 3 + 2 ≈ 0 . 2 6 1 0 5 2 3 8 4 4 4 0 1 0 3 1 8 3 0 9 6 8 1 2 5 .
By the way, the converse is also true. If, for some quadrilateral, the product of the measures of its diagonals is equal to the sum of the products of the measures of the pairs of opposite sides, then the quadrilateral is inscribable in a circle.