Who takes two language subjects?

We have 65% + 75% + 60% = 200%. Since none is taking three subjects then all of them are taking two subjects. Then the answer is no.

Consider the case when there are 20 students. Then 12 take math, 13 take French, and 15 take German. These numbers add up to 40, which is twice the actual number of students.

Let's imagine a student who takes French and math. This student counts towards the math and the French total, so the student is counted twice. Since the total is twice the number of students, that means that every student is counted twice, so every student takes two subjects. No student can be counted three times since no students take all three subjects.

This means there isn't a student who doesn't take French or German.

We can imagine a easiest way like we know there are 60% students take Mathematics, the best situation for Mathematics' student is the rest of students (40%) take both French and German.

Then there are still gonna be 25% for French and 35% for German, since none are taking all 3 subjects, there will only be French-Mathematics and German-Mathematics students = 25% + 35% = 60%

Thus, there aren't going to be a student who only take Mathematics = neither French nor German.

The answer is No

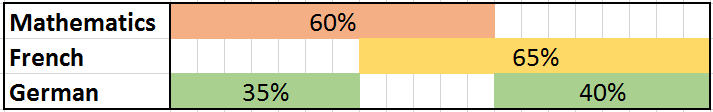
The only grouping possible for 60% of the students taking Mathematics, 65% taking French, 75% taking German and none taking all three subjects is as shown the figure above. That is: 35% taking Mathematics and German + 25% taking Mathematics and French + 40% taking Frech and German = 100%. Therefore, there is no student taking neither French nor German.

Here's a solution using a Venn-diagram: We know that: $M+X+Z = 60,$ $F+X+Y = 65,$ $G+Y+Z = 75,$ Which gives us : $M+F+G+2X+2Y+2Z = 200$ .

We also know that $M+F+G+X+Y+Z = 100$ so

$100+X+Y+Z = 200$

$X+Y+Z= 100$

That means $M+F+G = 0$

so $M = 0$

I looked at each course registered for by a student and created a table based on the problem assuming 100 students.

German 75

French 65

Math 60

Total 200

Students 100

Avg Courses 2

Since no student can register for more than 2 courses they can only register for 0 or 1 or 2.
Since the average number of courses registered for by the 100 students is 2, none can be anything other than 2, since any that take 0 or 1 would lower the average to less than 2.

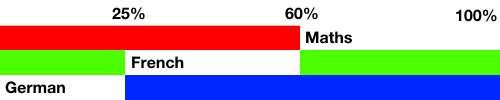Changing who takes French or German from this would result in students taking all three subjects.

Man it's so easy "group of students" if you don't study you are not a student. Then the answer is no

$\begin{cases} f+m+g+2(x+y+z) &= 200\% \\ f+m+g+x+y+z &= 100\% \end{cases}$

Subtracting the second equation from first gives $x+y+z = 100\%$ , which means that $m=0$ . Therefore, no student is taking math only.

Let there be 100 students in total . So 60 are take maths ,65 take French and 75 take German. Let there be one studen who is neither taking French nor German thus he has selected only maths. As there are 60 and 65 students in maths and French, at least 25 students have opted for both (60 +65 -100 =25) . So 75 students who opted for German must be the 75 students which are left (because there is no student who selected all three subjects) So there is no student who selected only maths.

If we create a Venn Diagram, and call the percentage of students that takes both Math and German $a$ , the percentage of students that take both Math and French $b$ and the percentage of students that take both German and French $c$ , the percentage of students taking Math only will be $60-a-b$ , the percentage of students taking German only will be $75-a-c$ and the percentage of students taking French only will be $65-b-c$ .

When we sum up all the values in the Venn diagram we end up with the total of the students, in this case it is 100 %.

$60-a-b+75-a-c+65-b-c=200-a-b-c=100$

Hence, $a+b+c=100$ .

If there are no students taking both German and French we have $c=0$ and $a+b=100$ . But if so, the percentage of students taking only Math will be $60-100=-40$ which is impossible!

Hence, we can conclude that must be some students that take both German and french and this number must be greater than 40% of the total.

Let's look at it another way, by inverting the percentages. We have 40% of the students not doing maths, and 35% not doing French. This means at least 25% of the students are doing both maths and French. By the same logic, at least 35% are doing both maths and German, and at least 40% doing both French and German. Since nobody is taking all three subjects, these three categories are disjoint. Hence, since the percentages add up to 100%, this means at least 100% of the students are taking two of the mentioned subjects. Since it can't be more than 100%, it must be exactly 100%. So every student is taking two of the three mentioned subjects. Ergo, nobody is taking neither French nor German. QED

To account for all students in the allowed combinations of two subjects only, some must take Maths and French (60% + 65% > 100%, so clearly some students take both), and some must take Maths and German (60% + 75% > 100%, so same story, some must take both). The case of students who take both French and German does not need to be considered in the context of the question. We can see that to account for all students, there is no possible solution that excludes both French and German.

The simplest solution to me seems to simply add people who take French and who take German, i.e. 65% + 75% = 140%. As this number is higher than 100%, there must be students who take both French and German.

65% are taking French, that leaves us with 35% who are not, In the best case all of them are taking German and out of the entire 75%, 40% are taking both. None of them can take mathematics so all the other 60% are taking Math. Let's assume not everyone who doesn't take French takes German than they must take all the 3 courses. In contradiction to the data.

Given 75% learn German so only 25% are not German students. Since 65% learn French, it is obvious that considerable number of students learn both.

Since French and German each exceed 50%, there must be students in each subject Ed Gray.