Wind powered upwind vehicle
Consider the plan below, which consists of a wheeled vehicle and a wind turbine that's connected to the wheels by nothing but a mechanical gear (there's no engine aboard). The inventor claims that it's a vehicle that can travel upwind, powered by the wind itself, and that it doesn't move if there's no wind.
Does this proposal violate either of the following principles and, if so, which one?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions
That's an interesting and wierd analogy. We can decompose the forces exerted by the screwdriver into a pure torque and an axial force. The torque will result in a screw moving outwards with some force, which allows the presence of some axial force of the screwdriver that is less that outward force of the screw. It's the same thing here, the wind provide the torque force, which will result in the vehicle moving into the wind, and "allow" for some axial wind resistance because the windmill and the vehicle doesn't have a negligible cross section.
Note: This is assuming that one uses a screwdriver in the normal way, which means twisting it as one pushes it against the screw! Otherwise, just pushing it doesn't do anything except to cause it seat itself more deeply into the hole, regardless if the screw is left or right handed.
Log in to reply
The wind does not provide torque unless it's blowing sideways. In that case you could get enough energy to compensate forward drag. But sailing boats already do that.
Log in to reply
The difference between typical axial or radial turbines and modern windmills is that the latter are designed with blades that are much more like wings of an aircraft. Just as there is a lift in the wings of an aircraft, there is a lift that provides torque to the axial rotor of the windmill. The key is that with windmills, the wind blows pretty much straight through, while with axial or especially radial turbines, airflow is deflected away from axial.
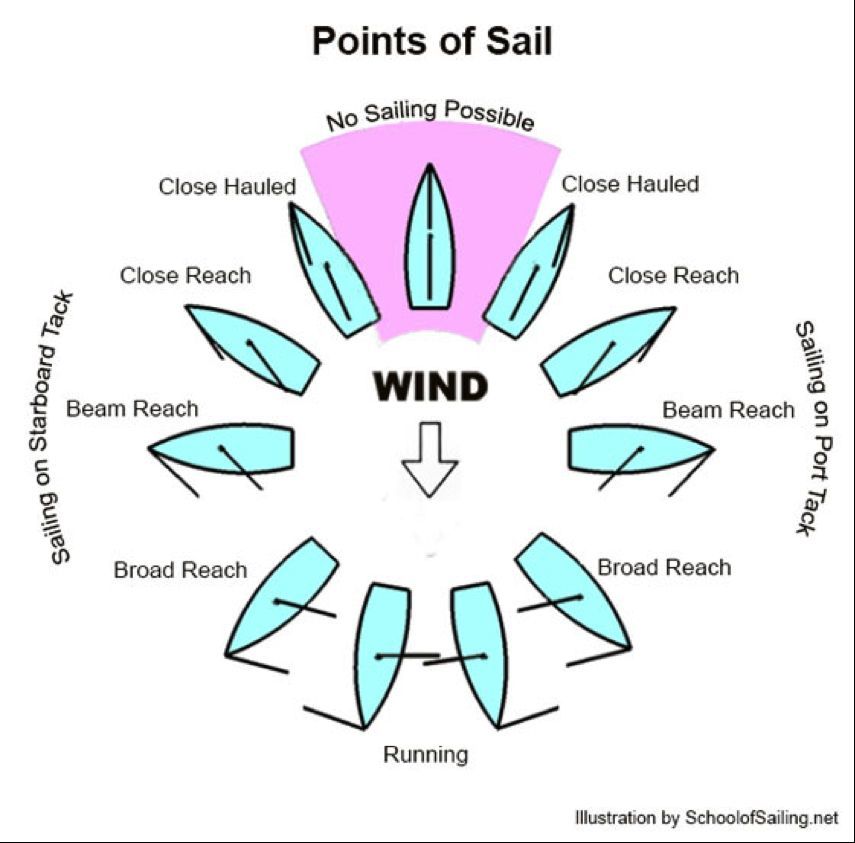
Conventional sailboats are not able to tack directly into the incoming wind. At that point, the sails are largely ineffective. The best they can do is to tack at some small angle away from the incoming wind.
You can't loosen a screw just by pushing forward. It would tight even more if there were no friction.
Log in to reply
The analogy for the system (my hand + screwdriver) is the system (wind + the turbine blades).
Except the screw driver isn't directly driving rotation of the screw threads. The screw driver is pushing the screw axially. This pushes the threads against the wood which it turn produce a component force on the screw threads. That component force which then turns the threads is less than the total force of the driver.
Newtons laws need to considered:
-
Energy can neither be conserved nor be destroyed but can only be transformed from one state to another. Since the cart would move only by the wind means wind energy would be transformed to kinetic energy. The cart would stop in the absence of wind. This would mean Energy is not transformed. Therefore there is no violation.
-
Every body continues to be in state of rest or continuous motion unless acted by an external force [disturbance]. Moving with the wind and stopping in the absence. Therefore there would not be a violation.
Won't the wind push against the vehicle building enough resistance to stop it?
The windmill can be designed such a way that it is powered mostly through lift normal to the direction of the wind. Then the energy collected in this way can be converted into mechanical power propelling the vehicle in any direction, including into the wind.
With the right kind of windmill blades, the axial force of the wind on the windmill can be small compared to the torque it could generate through blade lift.
It works amazingly well, both by land and by sea .
Log in to reply
That looks pretty cool, both of them. I'm surprised that even a homemade job can do it, as with the water craft. Let's see...I wonder if there's a way to modify the sails of a sailboat so that it can tack directly into the wind?
If it works, then as soon as the vehicle starts moving the apparent wind at the blades would increase and then more energy could be converted to power the vehicle, which would then generate more wind speed and so on... Perpetual motion?
Log in to reply
It will initially accelerate, but the turbine design will eventually reach its maximum efficiency.
Log in to reply
Suppose that the vehicle moves forward into the wind whose speed relative to the ground is 10, and the vehicle accelerates to a ground speed of 1. That means it is going 11 relative to the air. Let the wind die down by 1 and the vehicle's momentum causes it to continue at 1 relative to the ground. With the now wind speed of 9 plus the vehicle's ground speed of 1, we now have a relative wind of 10 again, which will accelerated the vehicle to 11 relative to the air or 2 relative to the ground. The wind dies down another 1 and the vehicle's momentum accelerates to maintain 11 relative to the air mass. Continuing this diminishing wind, we finally find the wind reaching 0 over the ground and the vehicle continuing at 11 relative to the still wind. NOW, we have perpetual motion.
What basis do you have for this maximum efficiency theorem?
Good answer.
It would be what u call a perpetual motion machine.. as far as I know that's only possible in theory
Log in to reply
Perpetual motion machines supposedly work from internal forces being recycled. This is a machine that works through an external force.
Log in to reply
The act of going forward in turn feeds the mechanism even more ... I think it's a classic example of PMM.
Log in to reply
@Lokesh Singh – It's specifically not "perpetual motion". The only concern is whether drag on the device is greater than the torque provided by the fan. Brian Charlesworth posted a video of a device just like this.
I also think so. In an ideal scenario the traction force would equal the wind drag and it wouldn't move. In practice I would expect it to move backwards due to inefficiencies in the power conversion and overall aerodynamic drag.
Can you explain what makes this a perpetual motion machine?
Log in to reply
I'm sure he's trying to say that if you took this vehicle out on a calm windless day, and towed it for a while until the windmill gets going, then it'll keep powering into the wind, going faster and faster, because the apparent wind will seem stronger. As he said, "that's only possible in theory."
Log in to reply
@Michael Mendrin – Precisely!!
Log in to reply
@Thomas Lippman – "that's only possible in theory"?
Yes!!! If the wind causes the vehicle to move into the wind, say 2 kt forward with 10 kts of wind. Then suppose the wind dies down to 9 kts. The vehicle still has 11 kts of relative wind (9 wind + 2 inertia), still enough to accelerate the vehicle by at least 2 kts, reaching 13 kts relative to the air mass. The wind dies down another kt as it typically does, leaving the vehicle still receiving 13 kts relative wind which will accelerate it another 2 kts at least. . . . . . . . . etc., etc.Eventually the vehicle will be traveling better than 10 kts over the ground with no wind at all. That's Perpetual motion!
If you are having difficulty accepting that it is only the relative wind that creates energy, replace the gearing between the turbine and wheels with a dynamo charging a battery and then compare the stationary vehicle facint a wind to a pickup truck pushing the vehicle at that same speed on a windless day. Can you see that whether the vehicle is moving through an air mass or an air mass moving over a stationary vehicle, the number of watts generated and the energy delivered and stored in the battery over an hour will be exactly the same? So then the question is why can't the energy stored in the battery be used to accelerate the vehicle whether the vehicle is moving or the air is moving? Doesn't Newton's formula F=MA apply equally to a moving vehicle as it does to a stationary vehicle?
The wind provides a constant supply of energy external to the vehicle. That makes it not a perpetual motion machine by definition.
The reason i think it violated the law of momentum is because the inventor claims that if there is no wind, the car doesn't move. Yet this is not true in the following case: The wind comes in hard blows and goes silent after the blows, when the car consumes this energy and starts moving forward, yet it keeps moving forward with the speed that it has gotten even though there is no wind for that moment, so that last claim about no wind no movement is technically false right?
Log in to reply
The vehicle will tend to keep moving forward once it has momentum, even if the wind were to temporarily die down. Think of a sailboat.
It says it won't move IF there's no wind, not that it won't move WHILE there's no wind.
This question did not give enough information
Log in to reply
The question means to ask if such a vehicle is possible at all without violating either of the conservation laws. The only source of energy available is from the wind itself.
It seems clear to me that if the force imparted by the wind on the turbine blade was sufficient to not only overcome the force exerted on the vehicle in the opposite direction. but to further accelerate it, then it follows that on a still day if you pushed the vehicle with your pickup truck, at the same speed that wind caused the vehicle to accelerate into the wind, the relative wind being the same, would accelerate the vehicle, leaving the pickup truck behind. This would be not only perpetual motion (a violation of the Second Law of Thermodynamics), but creating the force necessary to accelerate it originating from its own motion (a violation of the First Law of Thermodynamics).
Log in to reply
The wind turbine vehicle of the problem is continuously supplied with external energy by means of the wind which is driven by pressure differentials in the atmosphere due to a temperature gradient caused by solar energy.
Your pickup truck setup has no external energy being supplied to the vehicle of the problem. Initially, the pickup truck is burning gasoline to produce energy. Once the truck stops, there is no external energy source for the turbine. The vehicle grinds to a stop because the stored energy in the velocity of the vehicle is burned off by friction.
Log in to reply
But when the truck, and upwind vehicle (UV) being pushed, reach the speed that provides the vehicle with the same relative wind, the vehicle should accelerate just as it does when the wind blows and the vehicle was standing still. The only wind that the vehicle experiences is the relative wind. Any force manifested on the UV comes from that relative wind hitting the turbine. Whether the air mass moves and the UV is standing still, or the UV moves and the airmass is standing still, the relative wind is the same and therefore the force made available to the UV should be the same. Thus, the pushed UV, having the same relative wind and the same amount of force,should accelerate exactly as much as it did when the air mass movement created a relative wind as when pickup truck creates that relative wind. The pickup truck doesn't need to stop. It remains at constant speed while the vehicle accelerates and thus leaves the truck behind.
Log in to reply
@Thomas Lippman – What breaks the symmetry between the upwind motion of the problem, and your thought experiment with the pickup truck is that in one case there is relative motion between the wind and the ground, and in the other there isn't. That's the crux of what makes it all possible.
Log in to reply
@Josh Silverman – I do not understand how the wind relative to the ground makes any difference. Do you agree that it is the relative wind between the air mass and the vehicle that inputs power to the system in either case? If the relative wind is the same in either case, isn't the force or torque to the wheels the same also?
Did you also consider my argument with the turbine temporarily disconnected? Suppose we placed the vehicle pointed into the wind with the turbine disconnected from the wheels. The wind would turn the turbine freely but also push the vehicle downwind, because the vehicle itself is being acted upon by the wind. The vehicle would come close to but never reach the wind speed and the turbine, by then would barely be turning because the relative wind to the turbine is now near zero. So we engage the wheels to the turbine and since the wheels are going backward (downwind) the turbine will also turn backwards, causing the vehicle to continue going backward. From this it follows that we either have a bistable machine that can either go into the wind and accelerate or go downwind with its turbine accomplishing almost nothing. Can you think of a reason that the outcome equilibrium would be different depending on when the turbine is connected to the wheels?
My conclusion is that starting with the gearing engaged, the downwind force on the vehicle will exceed the force that can be delivered to the wheels in the upwind direction and the vehicle will move backwards. And if you change the gearing so that the upwind torque delivered to the wheels will exceed the downwind force, we must also consider that the mechanical advantage in force also requires a proportionate decrease in speed, in order for the power output to equal the power input, meaning that the speed upwind created by the relative wind will be slower than the speed of the vehicle going backwards, downwind. I maintain that the effect of the turbine will be to have a negligible effect on the vehicle's speed downwind.
Let us further consider a similar vehicle in the water (See https://www.youtube.com/watch?v=oKqC5JsurOk, which I believe is also fraudulent). Let's suppose that the turbine is connected directly to a propeller in the water and lets also suppose that the air is completely calm but the boat is sitting in a current moving in such a direction as to create a relative wind from the direction that the vehicle is facing. According to the theory initially proposed in the upwind vehicle, the relative wind acting on the turbine will produce forward motion in the vehicle such that the vehicle will move through the water faster than the water is moving over the ground, yes?. Does that sound possible? Doesn't it imply that the relative wind force will always have more impact on the turning of the turbine than it will on the backward motion of the vehicle, even if there were a large sail perpendicular to the relative wind erected above the turbine? Or is it possible that the vehicle will just move backward by virtue of the wind exerting more force on the vehicle and its sail than the turbine can possibly extract from the relative wind?
Log in to reply
I do not understand how the wind relative to the ground makes any difference.
Just to clarify, I wasn't suggesting that the mechanism should be obvious from this observation. All I'm saying is that, the two situations are not equivalent because no matter how you shift frames (e.g. by speeding up the buggy into the wind), you can never introduce a relative speed between the wind and ground by doing that. So there's a fundamental difference.
Do you agree that it is the relative wind between the air mass and the vehicle that inputs power to the system in either case?
I do, but given some velocity for the buggy, that relative wind speed depends on whether there is a wind (relative to the ground) or if the buggy is moving through still air. Note that the power that the wheels spend in pushing the vehicle depends on the speed of the buggy, but that isn't changed by there being a wind or not.
If the relative wind is the same in either case, isn't the force or torque to the wheels the same also?
No, this is where I depart.
Did you also consider my argument with the turbine temporarily disconnected? Suppose we placed the vehicle pointed into the wind with the turbine disconnected from the wheels. The wind would turn the turbine freely but also push the vehicle downwind, because the vehicle itself is being acted upon by the wind. The vehicle would come close to but never reach the wind speed and the turbine, by then would barely be turning because the relative wind to the turbine is now near zero. So we engage the wheels to the turbine and since the wheels are going backward (downwind) the turbine will also turn backwards, causing the vehicle to continue going backward. From this it follows that we either have a bistable machine that can either go into the wind and accelerate or go downwind with its turbine accomplishing almost nothing. Can you think of a reason that the outcome equilibrium would be different depending on when the turbine is connected to the wheels?
Actually, I don't disagree with this. The vehicle can operate in either mode ("upwind", or "downwind faster than the wind"), as long as you switch the gearing. In fact the land vehicle that @Brian Charlesworth posted a video of operates in both modes (as long as you switch the gearing around).
I have to run now but I'll be back tomorrow.
Log in to reply
@Josh Silverman – Let us break this down a bit further and rather than connecting the turbine directly to the wheels of the vehicle, let us connect the turbine to a dynamo or electric alternator charging a storage battery. Do you agree that the alternator will produce the very same power output, whether the vehicle is moving and the air mass standing still or the air mass is moving and the vehicle standing still? In other words, isn't it only the relative wind that determines whether or not and how much power can be generated by a turbine?.
Let's try another argument: Suppose we disengage the turbine from the vehicle's wheels. A wind blows against the front of the vehicle and the vehicle starts rolling downwind, while the disconnected turbine turns in the same direction to move the vehicle forward if it were connected, but now spins freely because there is no opposing load on the turbine. As the vehicle accelerates downwind, the relative wind on the turbine decreases. The vehicle never reaches the wind speed and the turbine never reaches zero wind speed. We then couple the turbine to the wheels. Three events are possible. The turbine will reverse from moving slowly and propel the vehicle forward despite its very slow speed with respect to the air mass. The vehicle will come to an abrupt stop. The vehicle's backward motion will turn the turbine backward and continue to go backward with the turbine now introducing a force in the downwind direction. The turbine now exerts a force moving the vehicle downwind with the exact same wind, same turbine and same gear ratio as it did when we supposed it would go forward into the wind. This sounds preposterous.
Here is another argument:
Let us consider a similar vehicle in the water (See https://www.youtube.com/watch?v=oKqC5JsurOk, which I believe is also fraudulent). Let's suppose that the turbine is connected directly to a propeller in the water and let's also suppose that the air is completely calm but the boat is sitting in a river where the current is moving in such a direction as to create a relative wind from the direction that the vehicle is facing. So the boat is moving forward over the ground but is still in the water. The air mass is still with respect to the ground but the boat is moving forward into the air mass, by virtue of the current.
According to the theory initially proposed in the upwind vehicle, the relative wind acting on the turbine will produce forward motion in the vehicle such that the vehicle will move forward through the water and faster over the ground than the water is moving over the ground, yes?. Does that sound possible? In the late afternoon, a gentle breeze begins in the same direction as the river's current, thus eliminating the relative wind that was caused by the current flow. The boat's velocity relative to the water as well as relative to the ground under the water now decreases with the addition of the new tailwind. That sounds preposterous. "The first principle is that you must not fool yourself and you are the easiest person to fool." - Richard P. Feynman, (1918-1988) US Physicist, teacher, musician
@Josh Silverman – Then we can look at this problem in two ways. 1. The wind acts on the turbine to turn it, which torque is transferred to the wheels which then propel the vehicle forward into the wind. 2. The wind acts on the vehicle producing a force that accelerates the vehicle in the same direction as the wind, causing the turbine to turn in the direction of adding to the force in the downwind direction, with no changing of the gearing.
Why do you like choice 1 better than choice 2? Is it only a matter of chance, which motion will occur? Does God actually shoot dice as Einstein refused to accept?
Suppose we placed the vehicle pointed into the wind with the turbine disconnected from the wheels. The wind would turn the turbine but also push the vehicle downwind, because the vehicle itself is being acted upon by the wind. The vehicle would come close to but never reach the wind speed and the turbine, by then would barely be turning because the relative wind is now near zero. So we engage the wheels to the turbine and since the wheels are going backward (downwind) and the turbine is hardly moving at all, the turbine will also turn backwards. From this it follows that we either have a bistable machine that can either go into the wind and accelerate or go downwind with its turbine accomplishing almost nothing.
My conclusion is that starting with the gearing engaged, the downwind force on the vehicle will exceed the force that can be delivered to the wheels in the upwind direction and the vehicle will move backwards. And if you change the gearing so that the upwind torque delivered to the wheels will exceed the downwind force, we must also consider that the mechanical advantage in force also requires a proportionate decrease in speed, meaning that the speed upwind created by the relative wind will be slower than the speed of the vehicle going backwards, downwind.
Log in to reply
Yes, the upwind speed of the vehicle will be less than the speed of the wind.
Energy is work which is force times distance. The gearing can be such that the distance the vehicle moves is very slow compared to the velocity of the wind.
We could go further in the analysis and determine the energy per unit time extracted from the wind and imparted on the vehicle but why bother. It is intuitively clear at this point that energy can be extracted from the wind at a rate that is sufficient to drive the vehicle slowly.
Log in to reply
The upwind speed of the vehicle may be less than the speed of the wind but relative to the wind, its velocity adds to the wind speed over the ground. If the vehicle accelerated from zero with a wind speed of 10, its relative wind then becomes 10 plus its ground speed. Keep in mind that the force derived from the wind is dependent on the relative wind, not the wind speed over the ground. To evaluate the vehicle's performance we have to stay in one frame of reference. Starting with the wind speed over the ground, switching to the wind hitting the turbine and then switching back to the vehicle's speed over the ground just confuses the matter. Start with the air mass relative to the vehicle and end with the air mass relative to the vehicle and it becomes clearer. If the air mass is moving at 10 kts relative to the vehicle, the force produced should be exactly the same as if the vehicle is moving 10 kts relative to the air mass and should produce the very same accelerative force on the vehicle relative to the air mass. Keeping in mind that a moving object requires no more force to maintain a constant velocity than a standing object. Any force introduced will accelerate the object.
If you employ a mechanical advantage to increase the force, you must sacrifice some speed in order to stay within the limitation of the First Law of Thermodynamics. To see that a force times a speed equalling Power must have the same inverse relationship as force times distance equalling work, just multiply both sides of the energy equality by time: Force times Speed times Time is, in fact Force times Distance.
I just corrected and clarified the last sentence to read "multiply both sides of the energy equality by time"
You haven't really explained the answer at all. Motion in the downwind direction (namely the wind) gets converted to motion in the upwind direction. How does this not violate conservation of momentum?
Log in to reply
We can look at physics like this in different ways, terms of energy, in terms of momentum, and also in terms of force. Here, I'm using force in explaining that the wind is exerting more force in terms of torque rather than axial if the windmill blades are designed right. Suppose the vehicle and the windmill had nearly zero cross-section area and therefore little resistance going into the wind, then it doesn't require a lot of torque force (converted mechanically) to impell it directly into the wind. I'll expand on my explanation later.
From an empirical point of view, it's not violating any conservation law because such a device is demonstrable.
Log in to reply
In order to explain it in terms of force, you would need to show that the forces in the axial direction can cancel each other out and still result in upwind motion of the vehicle. There must be downwind forces in the system that are sufficient to counterbalance (a) the absorption of the wind (b) the vehicle being driven upwind. These forces must be acting on something.
As for your final sentence, even if you can construct such a machine and demonstrate it working, doing so doesn't explain why it works.
Log in to reply
@Stewart Gordon – Well, then, how about if you posted a solution and explain 1) how it works, and 2) why it does not violate either of the two conservation laws? You do agree that it's demonstrable and therefore probably doesn't violate either of the laws.
The point that tripped me was that the problem stated, "It doesn't move if there's no wind." Suppose it is moving along and suddenly the wind stops. Shouldn't it keep moving under its momentum?
Conservation of energy and conservation of momentum are conserved within a system. In this case, the system is the vehicle and its immediate surrounding atmosphere. Since the energy and the force (and hence the momentum) is coming from outside the system (namely, from the wind), the vehicle doesn't necessarily violate neither of the two conservation principles.
EDIT: If you consider the wind part of the system, it still doesn't violate the conservation laws. Energy is still maintained as air molecules lose kinetic energy and the vehicle gains kinetic energy. Momentum is conserved, because although the vehicle moves towards the wind, the particles lose linear momentum to cause torque on the windmill and gears allow the torque to be increased and to be reversed in direction. The action of increasing and reversing the direction of a torque is akin to how a hydraulic press can increase and reverse the direction of the force applied. It does not violate the law of momentum and in fact is a result of the law of conservation of energy.
Let's try this. What if the vehicle has a cannonball interceptor that aborbs incoming cannonballs fired in its direction. Can it be made to travel forward towards the cannon firing them?
Log in to reply
It has to have a fan blade, doesn't it? Also I realised I only took into account angular momentum but not linear momentum, which is an error on my part.
Log in to reply
I actually hadn't figured out this scenario yet, I'm asking you. What if we made this as simple as possible, and just consider cannonballs shot towards the vehicle. Is there a way this vehicle can take mechanical advantage of it to go foward, without delecting the cannonball elsewhere? I.e., absorbing it, so that its mass increases? What could we then say about the conservation laws?
Log in to reply
@Michael Mendrin – Gears allow the angular velocity to be increased with the same amount of torque and also changes the direction of rotation. I think the cannon balls provide torque to the windmill, and that same amount of torque can be increased and reversed by the configuration of gears inside the vehicle, making it possible for it to move forward.
Log in to reply
@Johanan Paul – I'm saying, forget the windmill, take it out. Replace it with a box that takes inside cannonballs shot in its direction. Once inside the box, you can do anything you want with it to take advantage of its former kinetic energy, and convert it to the vehicle's forward motion, in the direction opposite of the incoming cannonballs. Is it even possible?
What happens to the conservation laws in this scenario?
Log in to reply
@Michael Mendrin – I think it might be possible theoretically. I'll assume energy is conserved and the transfer of energy is optimal. The scenario would look like a elastic collision, but instead of each object changing direction after collision, they maintain their same direction. In an elastic collision, the relative speed has to be maintained. So if the cannonball continues moving past the box but with a lower velocity (due to loss of KE), the vehicle has to move forward to maintain the relative speed.
My question is that, in this case, wind energy is being converted to mechanical energy to move the vehicle. As the vehicle moves faster, this wind in its direction becomes faster and hence more wind energy and more mechanical energy. Isn’t somehow the energy of the system getting increased.
Log in to reply
I you consider air not the be part of the system, then I can say that more air collisions occur per unit time, so the the power of energy that is transferred into the system is increased. And that energy that goes into the system isn't part of the system itself.
If you consider the air part of the system, then I can say increasing the speed creates a more efficient energy transfer from air as more collisions occur. But the total energy remains the same.
Furthermore, there will be opposing forces such as the friction with the ground and air resistance which would limit the speed. So energy won't be able to go on to infinity.
It does violate Conservation of Energy. If the wind provides enough energy to overcome the wind itself, it is creating more energy than it is collecting. This is best illustrated by pushing the vehicle on a windless day at the speed that the wind was claimed to be able to accelerate the vehicle into the wind. That being the case, if pushed at that speed, the turbine should also provide enough power to accelerate the vehicle away from the pushing vehicle, thus producing perpetual motion.
 It's actually quite easy to play with this design in Lego (and it works).
It's actually quite easy to play with this design in Lego (and it works).
The Amsterdam University of Applied Sciences also has a functional car on this principle.
This can be done even with LEGO? Wow.
The principle of conservation of momentum applies when there are no external forces on an object. So it simply doesn't apply here.
Whether the cart moves forward or backwards depends on the magnitude of V_wind as well as the efficiency of the gearing system.
Note that the third choice "It doesn't necessarily violate either principle" contains "necessarily". We can't say without more information whether a violation of energy occurs, so this is the correct answer.
Sailboats cannot go directly into the wind.
A ship can sail upwind so it must be possible
I think the solution might be to redesign the wheeled vehicle like a sailboat that moves upwind, then attach a vertical wind turbine.
They can't go directly upwind. Typically 40 degrees at best
I built one when I was about nine with tinker toys. It works.
Can we use it in real life?
This is not a solution, the physics was already explained by others. Just for fun:
Imagine you are using a screwdriver to loosen a tight screw. You are pushing the screwdriver but the screw moves backwards!