Wolfram Alpha can't calculate this limit, can you?
If n → ∞ lim ( ( 2 n 3 n ) ( 2 n 5 n ) ) n 1 can be expressed as q p , for coprimes p and q , what is the value of p + q ?
The answer is 3854.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
Same way...loved it .
Use Stirling's Approximation for this one, which leads to ( 5 5 n + 1 / 2 ⋅ 3 − 6 n − 1 ) 1 / n . As n → ∞ , we end up with 5 5 / 3 6 = 3 1 2 5 / 7 2 9 .
Hi Sir, You might wish to learn a bit of latex here
Amazingly, I didn't know that the problem was worth a few lines. The solution I thought of was this:
First , we expand both numerator and denominator to get :
q p = n → ∞ lim ( r = 1 ∏ 2 n ( n + r 3 n + r ) ) n 1
ln ( q p ) = n → ∞ lim n 1 r = 1 ∑ 2 n ln ( n + r 3 n + r )
⇒ ln ( q p ) = n → ∞ lim n 1 r = 1 ∑ 2 n ln ( 1 + r / n 3 + r / n )
⇒ ln ( q p ) = ∫ 0 2 ln ( 1 + x 3 + x ) d x = ln ( 7 2 9 3 1 2 5 )
⇒ q p = 7 2 9 3 1 2 5
Is this Sterling's approximation? If so, please tell how do we get the answer from it ?
Log in to reply
(cosmetic changes) Note that in your above statements, you cannot pull n 1 outside of the limit. Also, you're missing a d x in your integration.
Log in to reply
Thanks , edited!
Log in to reply
@Jatin Yadav – Since you are good at Calculus, the easiest way to derive Stirling's approximation is using gamma function .
Nice question and solution Jatin.
Calculus? I hope so.
n! can be approximated by √(2πn) (n/e)^n. I thought that was the easiest way to work this one out. Your solution is very interesting. Always great to look at different ways of solving the same problem.
Log in to reply
Thanks! Now, I understood how you use Sterling's approximation there! This problem is quite easy then.
Log in to reply
Sterling's approximation tends to be how people deal with limits involving n ! It tends to be the default substitution that is tried first.
 Use cauchy's 2nd limit theorem
Lim (un)^1/n = lim (un+1)/un
To get answer as 5^5/3^6
Use cauchy's 2nd limit theorem
Lim (un)^1/n = lim (un+1)/un
To get answer as 5^5/3^6
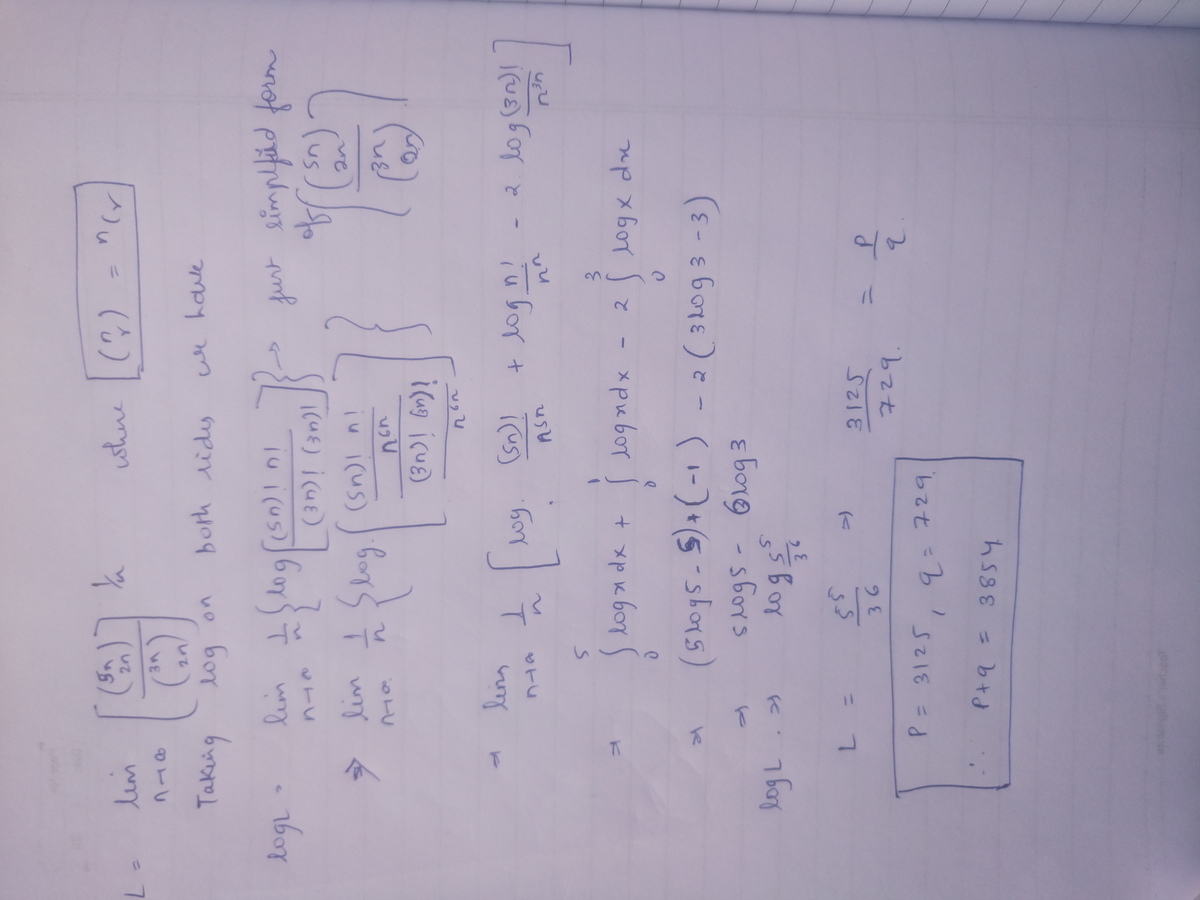
Stirling's approximation seem to be the best approach, and maybe Riemann sum. Here's another approach :
Set a n to be the thing inside the big brackets, so : a n a n + 1 = 9 ( 1 + 3 n ) 2 ( 2 + 3 n ) 2 5 ( 5 n + 1 ) ( 5 n + 2 ) ( 5 n + 3 ) ( 5 n + 4 ) → 3 6 5 5 = 7 2 9 3 1 2 5 = l . By Stolz lemma we get n a n → l which gives the answer.